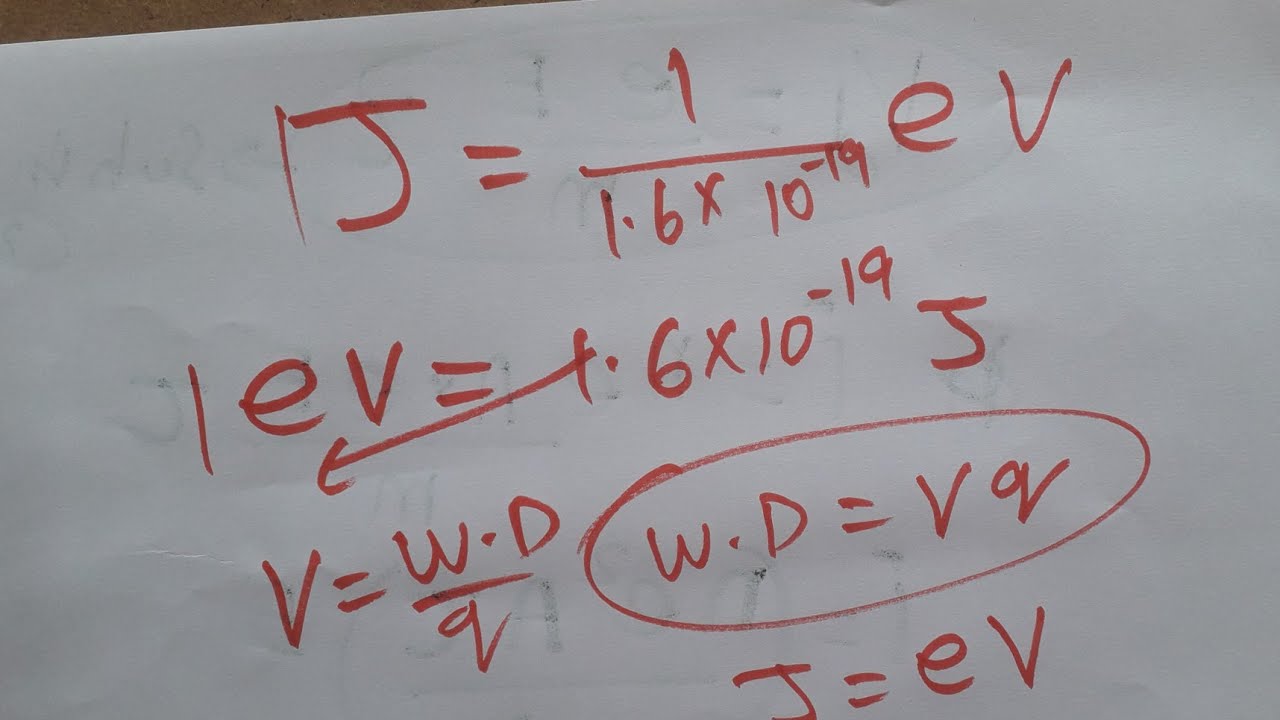Joules to eV: Conversion Guide & Applications

The conversion from joules to eV is fundamental in fields ranging from semiconductor physics at institutions like MIT, where energy band calculations rely heavily on understanding electron behavior, to the development of cutting-edge technologies by organizations such as CERN, where particle energies are often measured in electronvolts. The National Institute of Standards and Technology (NIST) provides essential constants and conversion factors that facilitate accurate joules to eV calculations, which are used extensively in software tools like OriginPro for data analysis and visualization in energy-related experiments. Energy in joules, a standard SI unit, represents macroscopic work, while the electronvolt (eV) conveniently quantifies the energy of individual particles at the atomic level.

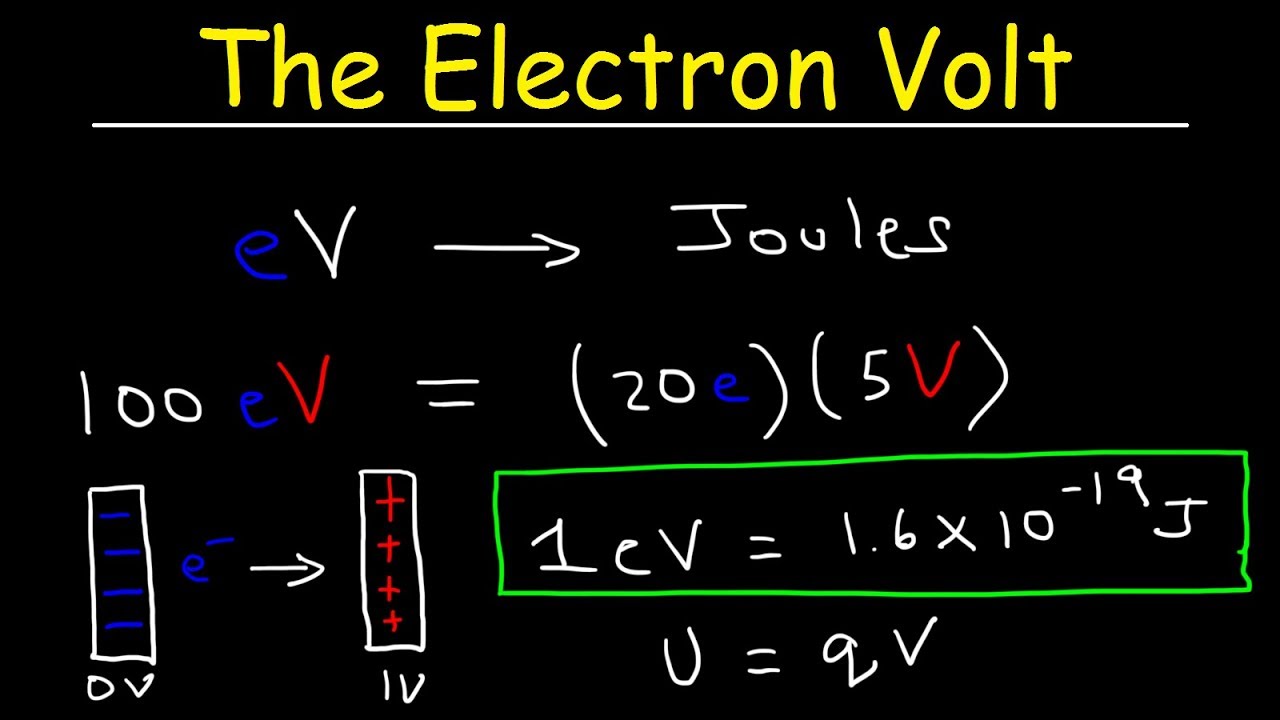
Image taken from the YouTube channel The Organic Chemistry Tutor , from the video titled Electron Volt Explained, Conversion to Joules, Basic Introduction .

Bridging the Worlds of Joules and Electronvolts: An Essential Conversion

At the heart of physics lies the concept of energy, the fundamental capacity to do work. Energy manifests in countless forms, from the kinetic energy of a moving object to the potential energy stored in a chemical bond.

The measurement and quantification of energy are paramount to understanding and manipulating the physical world. To that end, we rely on units – standardized measures that provide a common language for expressing energetic quantities.

The Importance of Units in Physics

Units provide a consistent and reproducible framework for scientific communication. Without universally accepted units, comparing experimental results and theoretical predictions would be impossible.

Imagine trying to describe the energy released in a nuclear reaction if each scientist used their own arbitrary scale. Chaos would ensue!

Introducing the Joule: The SI Unit of Energy

The Joule (J), named after the physicist James Prescott Joule, is the standard unit of energy in the International System of Units (SI). It is defined as the amount of work done when a force of one newton displaces an object a distance of one meter in the direction of the force.

The Joule is a versatile unit, applicable across a wide range of energy scales, from macroscopic mechanical systems to microscopic thermal processes.

Introducing the Electronvolt: A Practical Unit for the Infinitesimal

While the Joule reigns supreme in many domains, another unit, the electronvolt (eV), emerges as particularly useful when dealing with energies at the atomic and subatomic levels.

One electronvolt is defined as the amount of energy gained (or lost) by a single electron when it moves through an electric potential difference of one volt.

This unit conveniently scales to the typical energies encountered in atomic and particle physics, where Joules would often involve cumbersome exponents. Think of it as using kilometers instead of millimeters when measuring the distance between cities.

Why Understand the Joule-to-Electronvolt Conversion?

The realms of macroscopic phenomena (often described in Joules) and microscopic phenomena (often described in electronvolts) are not separate and insulated. Instead, they are intimately connected. Understanding the relationship between these units is critical.

For example, calculating the energy required to ionize an atom (typically expressed in eV) and then using that atom to power a macroscopic device requires a conversion to Joules.

Furthermore, scientists and engineers frequently encounter data expressed in either Joules or electronvolts. To effectively interpret and apply this information, a clear understanding of the conversion between these units is essential.

A Comprehensive Guide to Joule-Electronvolt Conversion

This article serves as a comprehensive guide to converting between Joules and electronvolts. We will delve into the precise conversion factor, provide step-by-step instructions, and illustrate the conversion process with practical examples.

Whether you are a student grappling with atomic physics or a seasoned researcher exploring new materials, this guide will equip you with the knowledge and skills necessary to navigate the energetic landscape with confidence.

Understanding the Fundamentals: Joules and Electronvolts Defined

Before delving into the conversion process, it's crucial to establish a solid understanding of the units involved: the Joule (J) and the electronvolt (eV). These units, while both measuring energy, arise from different contexts and scales, making their individual definitions essential for grasping their interrelation.

The Joule (J): Energy in the Macrocosm

The Joule, named after the English physicist James Prescott Joule, is the fundamental unit of energy within the International System of Units (SI). Its definition stems directly from the concepts of work and force. One Joule is defined as the amount of work done when a force of one Newton is applied over a distance of one meter.

This can be expressed mathematically as:

1 J = 1 N⋅m = 1 kg⋅m²/s²

This definition firmly anchors the Joule within the macroscopic world, relating it to everyday experiences of force, distance, and mass. It's the unit we use to measure the energy consumption of appliances, the kinetic energy of a moving car, or the potential energy of an object held at a height.

The Electronvolt (eV): Energy in the Quantum Realm

The electronvolt, in contrast, emerges from the microscopic world of atoms and particles. It is defined as the amount of kinetic energy gained by a single electron when it accelerates through an electric potential difference of one volt.

This definition directly links the electronvolt to the elementary charge (e), the fundamental unit of electric charge carried by a single proton or electron.

The relationship is:

1 eV = e × 1 V

Where e ≈ 1.602176634 × 10⁻¹⁹ Coulombs (C).

The electronvolt provides a convenient and intuitive way to express energies at the atomic and subatomic levels. Consider, for instance, the energy levels of electrons within an atom or the kinetic energies of particles in a particle accelerator. Expressing these energies in Joules would involve exceedingly small numbers, making the electronvolt a far more practical choice.

Why eV is Convenient for Atomic-Scale Energies

The convenience of the electronvolt stems from the scale of energies encountered in atomic and subatomic phenomena. Energies at this scale are typically on the order of a few electronvolts. Using Joules would result in extremely small numbers, making calculations and comparisons cumbersome.

Imagine describing the ionization energy of hydrogen (the energy required to remove an electron from a hydrogen atom). In Joules, it's approximately 2.18 × 10⁻¹⁸ J. In electronvolts, it's a much more manageable 13.6 eV.

This difference in scale highlights the practical advantage of the electronvolt in the realm of atomic and particle physics.

The Significance of Energy Measurement

The ability to accurately measure energy is paramount across a wide spectrum of scientific disciplines. From determining the efficiency of energy conversion processes to understanding the fundamental interactions of particles, energy measurements provide critical insights.

In chemistry, for instance, bond energies, reaction enthalpies, and activation energies are all essential parameters measured in Joules or electronvolts. In biology, the energy content of food (measured in Joules or calories, which are directly related to Joules) is crucial for understanding metabolism and nutrition. In engineering, the energy efficiency of machines and power plants is a key design consideration.

Ultimately, the accurate measurement and understanding of energy, whether expressed in Joules or electronvolts, underpins much of our scientific and technological progress.

The Magic Number: Unveiling the Joule-to-eV Conversion Factor

Understanding the relationship between Joules and electronvolts hinges on a single, crucial value: the conversion factor. This "magic number" acts as the bridge, enabling us to translate energy measurements from the macroscopic world (Joules) to the atomic and subatomic realms (electronvolts), and vice versa.

The Precise Value

The conversion factor between Joules and electronvolts is:

1 eV = 1.602176634 × 10^-19 Joules

This value is fundamental to calculations in numerous scientific disciplines, particularly in contexts involving charged particles and electromagnetic interactions. It is imperative that this value is used accurately for precision.

Deriving the Conversion Factor: A Closer Look

The conversion factor isn't arbitrary; it stems directly from the definition of the electronvolt. Remember that an electronvolt is defined as the amount of kinetic energy gained by a single electron when it accelerates through an electric potential difference of one volt.

This brings us to the elementary charge (e), which is the magnitude of the electric charge carried by a single proton or electron. The accepted value of the elementary charge is:

e = 1.602176634 × 10^-19 Coulombs (C)

When an electron with this charge moves through a potential difference of one volt (1 V), the work done on the electron (and hence the energy it gains) is given by:

Energy = Charge × Potential Difference

Substituting the value of the elementary charge and the potential difference of 1 V, we get:

Energy = (1.602176634 × 10^-19 C) × (1 V) = 1.602176634 × 10^-19 Joules

Therefore, 1 eV is equal to 1.602176634 × 10^-19 Joules, which is the conversion factor we use.

The Importance of Accurate Values and Reliable Sources

The precision of the Joule-to-eV conversion directly relies on the accuracy of the elementary charge value. Science and engineering communities, rely on authoritative sources, such as the National Institute of Standards and Technology (NIST), for this value.

NIST regularly updates its physical constants based on the latest experimental data and theoretical refinements. Using outdated or inaccurate values can lead to significant errors in calculations, especially when dealing with extremely small or large energy scales.

Always consult reliable sources like NIST, the Particle Data Group (PDG), or peer-reviewed scientific literature to ensure you're using the most up-to-date and accurate value for the elementary charge and, consequently, the Joule-to-eV conversion factor. This diligence ensures the integrity and reliability of scientific results.

Conversion in Action: A Practical Guide to Mastering Joule-to-eV Conversions

[The Magic Number: Unveiling the Joule-to-eV Conversion Factor Understanding the relationship between Joules and electronvolts hinges on a single, crucial value: the conversion factor. This "magic number" acts as the bridge, enabling us to translate energy measurements from the macroscopic world (Joules) to the atomic and subatomic realms...]

Now, let's put this knowledge into practice. Converting between Joules and electronvolts doesn't have to be intimidating. This section provides a clear, step-by-step guide to performing these conversions with confidence, ensuring you can seamlessly navigate between these units.

Converting Joules to Electronvolts: A Step-by-Step Approach

The fundamental principle behind converting Joules to electronvolts lies in understanding how many electronvolts are equivalent to one Joule. The conversion is straightforward, requiring a simple division.

The key formula is:

eV = Joules / (1.602176634 × 10^-19)

This formula essentially answers the question: how many units of 1.602176634 × 10^-19 Joules (the energy equivalent of 1 eV) are contained within the given number of Joules?

Example Calculation: Converting 5 Joules to eV

Let's say we want to convert 5 Joules to electronvolts. We simply apply the formula:

eV = 5 J / (1.602176634 × 10^-19 J/eV)

eV ≈ 3.12 × 10^19 eV

Therefore, 5 Joules is approximately equal to 3.12 × 10^19 electronvolts.

Converting Electronvolts to Joules: Bridging the Atomic to the Macroscopic

Converting from electronvolts to Joules is the inverse operation, allowing us to express atomic-scale energies in the more familiar unit of Joules.

The governing formula is:

Joules = eV × (1.602176634 × 10^-19)

This formula effectively scales the energy value expressed in electronvolts to its equivalent in Joules, taking into account that 1 eV = 1.602176634 × 10^-19 J.

Example Calculation: Converting 10 eV to Joules

Consider converting 10 eV to Joules. Using the formula:

Joules = 10 eV × (1.602176634 × 10^-19 J/eV)

Joules ≈ 1.602 × 10^-18 J

Thus, 10 electronvolts is approximately equal to 1.602 × 10^-18 Joules.

Dimensional Analysis: Your Key to Accurate Conversions

While the formulas themselves are quite simple, maintaining accuracy requires careful attention to units. This is where dimensional analysis comes into play.

Dimensional analysis involves treating units as algebraic quantities that can be multiplied, divided, and canceled. By tracking units throughout the calculation, you can ensure that the final answer has the correct units.

For example, in converting Joules to eV, you are essentially dividing Joules by Joules/eV. The Joules units cancel out, leaving you with eV.

Conversely, in converting eV to Joules, you are multiplying eV by Joules/eV. The eV units cancel, leaving you with Joules.

A thorough understanding of dimensional analysis prevents errors, especially when dealing with complex calculations involving multiple unit conversions.

Always double-check that the units in your answer make logical sense, and that they are what you expect based on the conversion you're performing. This simple habit can save you from making costly mistakes.

eV in Particle and Atomic Physics: Illuminating the Infinitesimal

Having established the methodology for converting between Joules and electronvolts, it's time to explore the practical applications of the electronvolt, particularly in the realms of particle and atomic physics. Here, where energy scales are often exceedingly small, the electronvolt emerges as not just a convenient unit, but an indispensable tool for understanding the fundamental building blocks of our universe.

The Electronvolt in Particle Accelerators

In the high-energy world of particle physics, the electronvolt and its multiples—keV, MeV, GeV, and TeV—become the lingua franca for expressing particle energies. When physicists discuss the Large Hadron Collider (LHC) at CERN, they speak of collision energies reaching 13 TeV. This energy, equivalent to the kinetic energy of two mosquitoes colliding, is concentrated within a space smaller than a proton, enabling groundbreaking discoveries about the nature of matter and fundamental forces.

The electronvolt scale allows for a manageable representation of the immense energies involved in these collisions. Imagine trying to express a TeV (10^12 eV) in Joules directly; the sheer magnitude of the number becomes unwieldy and obscures the relative energy scales.

Atomic Energy Levels and Ionization

Moving down the energy spectrum, the electronvolt plays a crucial role in describing the behavior of atoms and molecules. The energies associated with electron transitions between different energy levels are typically on the order of electronvolts. When an electron jumps from a higher energy level to a lower one, it emits a photon with an energy corresponding to the difference between the two levels, expressed in eV.

These energy differences are unique for each element, forming the basis of atomic spectroscopy. Similarly, the ionization energy, the energy required to remove an electron from an atom, is also conveniently measured in electronvolts. For example, the ionization energy of hydrogen is 13.6 eV, a value that resonates throughout atomic physics.

Band Gaps and Impurities in Solid-State Physics

In the realm of solid-state physics and semiconductor physics, the electronvolt helps to characterize the electronic properties of materials. The band gap, a critical parameter determining the conductivity of a semiconductor, is typically measured in eV. For instance, silicon, a cornerstone of modern electronics, has a band gap of approximately 1.1 eV.

The intentional introduction of impurities, or doping, into semiconductors alters their electronic behavior by creating energy levels within the band gap. These impurity energy levels, also measured in electronvolts, dictate the type and concentration of charge carriers, enabling the fabrication of transistors and other essential electronic components.

Nuclear Binding Energy and Reactions

Stepping into the nucleus, we encounter another domain where the electronvolt proves invaluable. The nuclear binding energy, the energy required to disassemble a nucleus into its constituent protons and neutrons, is typically on the scale of MeV (million electronvolts). This enormous energy reflects the strong nuclear force that binds nucleons together against the repulsive electromagnetic force between the positively charged protons.

Nuclear reactions, such as nuclear fission and fusion, involve the release or absorption of energy, again conveniently expressed in MeV. The energy released in nuclear fission, the process used in nuclear power plants, is on the order of 200 MeV per fissioned nucleus.

Kinetic and Potential Energy: Choosing the Right Scale

Even in scenarios involving kinetic and potential energy, the electronvolt can be a preferred unit. For example, when analyzing the motion of electrons in a vacuum tube or the behavior of ions in a plasma, expressing their energies in electronvolts often provides a more intuitive understanding than using Joules.

If we think of an electron accelerated through a potential difference of 1 Volt, it gains a kinetic energy of 1 eV. This direct relationship simplifies calculations and provides a clear visualization of the energy involved.

In summary, the electronvolt is more than just a unit of energy; it is a lens through which we can view and understand the intricate workings of the universe at its most fundamental levels. From the energies of colliding particles to the binding energies within atomic nuclei, the electronvolt illuminates the infinitesimal.

Beyond Physics: Applications of eV in Diverse Scientific Fields

Having established the methodology for converting between Joules and electronvolts, it's time to explore the practical applications of the electronvolt, particularly in the realms of particle and atomic physics. Here, where energy scales are often exceedingly small, the electronvolt emerges not just as a convenient unit, but as an indispensable tool for understanding the microcosm. However, the utility of the eV extends far beyond the conventional boundaries of physics, permeating various scientific disciplines. It is crucial to understand its use across different fields to truly grasp its importance.

Electronvolts in Spectroscopy: Probing the Electromagnetic Spectrum

Spectroscopy, the study of the interaction between matter and electromagnetic radiation, heavily relies on the concept of photon energy.

The energy of a photon is directly proportional to its frequency and inversely proportional to its wavelength, as described by the equation E = hν = hc/λ, where E is energy, h is Planck's constant, ν is frequency, c is the speed of light, and λ is wavelength.

Expressing photon energies in electronvolts is particularly convenient when dealing with different regions of the electromagnetic spectrum.

For instance, ultraviolet (UV) photons typically have energies in the range of a few to tens of eV, while visible light photons fall within the 1.6 eV to 3.3 eV range. Infrared (IR) photons possess even lower energies, often less than 1.6 eV.

This allows researchers to easily compare and contrast the energies of photons across different spectral regions and their corresponding effects on matter.

Plasma Physics: Gauging the Temperature of Ionized Gases

In plasma physics, where matter exists in an ionized state, the electronvolt serves as a natural unit for characterizing electron temperatures and energies.

Plasma temperatures are often expressed in terms of the average kinetic energy of the electrons, and converting this energy to electronvolts provides a readily understandable measure.

For example, a plasma with an electron temperature of 1 eV signifies that the average kinetic energy of the electrons is equivalent to the energy gained by a single electron accelerating through a potential difference of 1 volt.

This simplifies calculations and comparisons in plasma research, aiding in the development of fusion energy, plasma processing, and space propulsion technologies.

Materials Science: Quantifying Atomic Binding Energies

Materials science benefits significantly from the use of electronvolts in quantifying the binding energies of atoms within crystal lattices. These binding energies determine the stability and properties of materials.

The energy required to remove an atom from its position in the lattice is often expressed in electronvolts, providing a direct measure of the strength of the interatomic bonds.

Understanding these binding energies is crucial for designing new materials with desired mechanical, thermal, and chemical properties. From high-strength alloys to advanced ceramics, the eV plays a vital role.

X-ray Technology: Defining Photon Energies for Imaging and Therapy

X-ray technology, encompassing both medical imaging and industrial inspection, relies on precisely controlled X-ray photon energies.

The energy of X-ray photons, typically ranging from keV to MeV, determines their penetration power and interaction with matter. Expressing these energies in electronvolts allows for precise control over the imaging process.

It also enables optimization of radiation doses in medical applications, ensuring that patients receive the necessary diagnostic or therapeutic benefit while minimizing exposure to harmful radiation.

Radiation Therapy: Delivering Precise Energy Doses

In radiation therapy, the accurate delivery of energy to cancerous tissues is paramount. The energies of radiation beams used in cancer treatment are carefully calibrated in electronvolts.

These beams, consisting of photons, electrons, or ions, deposit their energy within the tumor cells, disrupting their DNA and preventing them from replicating.

The choice of beam energy, expressed in MeV or GeV, depends on the depth and location of the tumor, as well as the surrounding healthy tissues.

Precise energy control, facilitated by the electronvolt scale, is essential for maximizing therapeutic efficacy while minimizing damage to healthy organs.

Photovoltaics: Optimizing Solar Cell Efficiency

Photovoltaics, the science of converting sunlight into electricity, relies heavily on understanding the band gaps of semiconductor materials used in solar cells.

The band gap, expressed in electronvolts, represents the minimum energy required to excite an electron from the valence band to the conduction band, enabling the flow of electrical current.

The efficiency of a solar cell is directly related to the band gap of its constituent materials. Optimal band gap values, typically around 1.1 eV to 1.7 eV for silicon-based solar cells, allow for efficient absorption of sunlight and generation of electricity.

Advancements in photovoltaic technology are driven by the development of new materials with tailored band gaps, maximizing energy conversion efficiency and reducing the cost of solar energy. The eV, thus, underpins sustainable energy development.

Video: Joules to eV: Conversion Guide & Applications

Play Video

So, whether you're knee-deep in quantum physics or just trying to wrap your head around energy units, hopefully, this guide has helped demystify the whole joules to eV conversion thing. Now you can confidently switch between joules and electronvolts, and maybe even impress your friends with your newfound knowledge!