Antoine Equation: Vapor Pressure Made Easy

The Antoine equation, a semi-empirical correlation, provides a practical method for estimating the vapor pressure of pure substances. This mathematical model, widely utilized in chemical engineering, relies on constants specific to each compound, often found in databases like the NIST Chemistry WebBook. Its utility extends to practical applications, such as process simulation using software like Aspen Plus, where accurate vapor pressure data is crucial for design and optimization. Developed by the French scientist Louis Charles Antoine, the equation simplifies complex thermodynamic relationships into a user-friendly format for predicting vapor pressure at various temperatures.

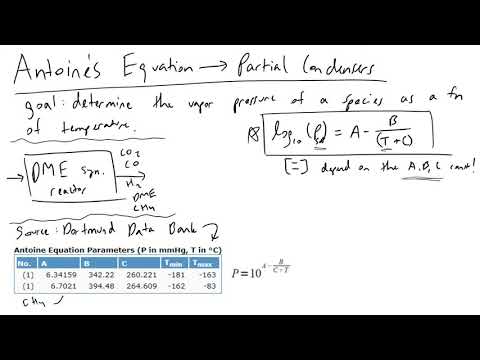
Image taken from the YouTube channel Vincent Stevenson , from the video titled Antoine's Equation Intro and Example .

The Antoine equation stands as a practical tool for estimating vapor pressure, a crucial property in thermodynamics and chemical engineering.

This empirical equation provides a relatively simple yet effective method for approximating the vapor pressure of pure substances as a function of temperature. This section introduces the concept of vapor pressure, its significance, and how the Antoine equation offers an empirical approach to its determination.

Understanding Vapor Pressure

Vapor pressure is defined as the pressure exerted by a vapor in thermodynamic equilibrium with its condensed phases (solid or liquid) at a given temperature in a closed system. It is a measure of the tendency of a substance to evaporate or sublimate.

The higher the vapor pressure at a given temperature, the greater the rate of evaporation of the liquid. Vapor pressure is crucial in many areas of science and engineering.

Significance in Thermodynamics and Chemical Engineering

Vapor pressure plays a critical role in various applications. This includes distillation, evaporation, drying, and other separation processes.

It is also vital for understanding phase behavior, predicting reaction kinetics, and designing chemical reactors. Understanding vapor pressure is essential for ensuring the safety and efficiency of industrial processes.

Vapor Pressure and Saturation Vapor Pressure

Vapor pressure is closely related to saturation vapor pressure, which represents the maximum vapor pressure a substance can achieve at a specific temperature.

When the vapor pressure equals the saturation vapor pressure, the system is in phase equilibrium. This means the rate of evaporation equals the rate of condensation.

This equilibrium is fundamental to understanding phase transitions and predicting the behavior of mixtures.

The Antoine Equation: An Empirical Approach

The Antoine equation is an empirical formula used to estimate the vapor pressure of a pure substance as a function of temperature.

The equation is expressed as:

log₁₀(P) = A − (B / (T + C))

Where:

• P is the vapor pressure
• T is the temperature
• A, B, and C are Antoine coefficients, which are specific to each substance

Structure and Purpose

The Antoine equation provides a straightforward way to calculate vapor pressure using substance-specific constants and temperature. It is an empirical formula, meaning that it is based on experimental data rather than derived from first principles.

This makes it a practical tool for engineers and scientists who need to quickly estimate vapor pressures without complex calculations.

The A, B, and C constants are crucial components of the Antoine equation. These constants are determined experimentally for each substance and reflect the unique molecular properties that influence its vapor pressure behavior.

Values for these constants can be found in various chemical handbooks, databases, and online resources. It is essential to use reliable and validated coefficient data to ensure accurate vapor pressure estimations.

An empirical equation, such as the Antoine equation, is based on observation and experimental data rather than theoretical derivations.

While empirical equations can provide accurate results within the range of data used to derive them, they have limitations. They may not be accurate when extrapolated beyond the range of experimental data or applied to substances with significantly different properties.

Users should be aware of these limitations and validate results with experimental data or more rigorous thermodynamic models when necessary.

Louis Charles Antoine (1825-1898) was a French engineer who developed the equation in 1888, as a simplified form of the more complex Clausius-Clapeyron relation. His work provided a practical tool for estimating vapor pressures, which has been widely used in chemical engineering and related fields.

Key Components and Variables of the Antoine Equation

The Antoine equation stands as a practical tool for estimating vapor pressure, a crucial property in thermodynamics and chemical engineering. This empirical equation provides a relatively simple yet effective method for approximating the vapor pressure of pure substances as a function of temperature. This section introduces the concept of vapor pressure and details the essential components that drive the Antoine equation: temperature and vapor pressure.

Temperature: The Driving Force

Temperature stands as the independent variable within the Antoine equation, exerting a powerful influence on a substance's vapor pressure. As temperature increases, the kinetic energy of molecules rises, allowing more of them to escape from the liquid phase into the gaseous phase, thus elevating the vapor pressure. This relationship is fundamental to understanding phase transitions and chemical processes.

Temperature Units and Conversions

The Antoine equation accommodates various temperature scales, with Celsius (°C) and Kelvin (K) being the most prevalent. However, the constants A, B, and C are specific to the temperature scale used, requiring careful attention to units.

• Celsius (°C): Often used in experimental data and readily available in many references.

• Kelvin (K): The absolute temperature scale, where K = °C + 273.15. Using Kelvin ensures thermodynamic consistency in many calculations.

Careful conversion between these units is essential to avoid significant errors in vapor pressure estimations. Always check the reference source for the Antoine coefficients to confirm the correct temperature scale.

Vapor Pressure: The Dependent Variable

Vapor pressure emerges as the dependent variable in the Antoine equation, representing the pressure exerted by a substance in its gaseous phase at a given temperature. It is the equilibrium pressure achieved when the rate of evaporation equals the rate of condensation in a closed system.

Units of Vapor Pressure and Their Implications

The Antoine equation can yield vapor pressure in various units, including:

• mmHg (millimeters of mercury): A traditional unit, still found in many legacy datasets.

• kPa (kilopascals): The SI unit of pressure, providing a more standardized representation.

  You also like

  Playing Video Games Sweating: Causes & Fixes

• bar: Commonly used in engineering applications, with 1 bar approximately equal to atmospheric pressure.

The choice of units often depends on the specific application and the source of the Antoine coefficients. Regardless of the unit, the vapor pressure value provides critical information about a substance's volatility and its behavior in different environments.

Practical Significance of Vapor Pressure Values

The magnitude of vapor pressure has significant practical implications. Substances with high vapor pressures at ambient temperatures are considered volatile, readily evaporating into the air. This characteristic is crucial in applications ranging from perfume formulation to the design of chemical reactors. Conversely, substances with low vapor pressures are less prone to evaporation, making them suitable for applications where containment and stability are paramount.

Theoretical Underpinnings: Connecting to Thermodynamics

The Antoine equation stands as a practical tool for estimating vapor pressure, a crucial property in thermodynamics and chemical engineering. This empirical equation provides a relatively simple yet effective method for approximating the vapor pressure of pure substances as a function of temperature. However, to fully appreciate its utility and limitations, it's crucial to understand its theoretical roots and how it relates to more fundamental thermodynamic principles.

The Clausius-Clapeyron Connection

At its core, the Antoine equation is a simplified, empirical manifestation of a more fundamental thermodynamic relationship: the Clausius-Clapeyron equation. The Clausius-Clapeyron equation provides a rigorous description of the relationship between vapor pressure and temperature based on thermodynamic principles.

It's derived from the first and second laws of thermodynamics and incorporates the concept of enthalpy of vaporization. This equation offers a more theoretically sound approach to understanding vapor pressure behavior.

The Antoine equation, on the other hand, is an empirical formula developed by fitting experimental vapor pressure data to a specific mathematical form. While it lacks the rigorous thermodynamic derivation of the Clausius-Clapeyron equation, it offers a convenient and computationally efficient way to estimate vapor pressures.

Differences and Similarities

The primary difference lies in their derivation and generality. The Clausius-Clapeyron equation is derived from fundamental thermodynamic principles. It is applicable to a wide range of substances and conditions, although it often requires simplifying assumptions to be integrated.

The Antoine equation, by contrast, is entirely empirical. It relies on experimentally determined constants (A, B, and C) that are specific to each substance. This means it is only valid within the temperature range for which the constants were determined.

However, the Antoine equation can be seen as an approximation or simplification of the integrated Clausius-Clapeyron equation under certain assumptions. Both equations capture the exponential relationship between vapor pressure and temperature, reflecting the underlying thermodynamic principles governing phase equilibrium.

Boiling Point Significance

The boiling point of a liquid is intrinsically linked to its vapor pressure. The boiling point is defined as the temperature at which the vapor pressure of the liquid equals the surrounding atmospheric pressure. At this temperature, the liquid readily transitions into the gaseous phase.

The Antoine equation can be a valuable tool for estimating boiling points. By setting the vapor pressure in the equation equal to the desired pressure (e.g., atmospheric pressure, which is typically 1 atm or 760 mmHg) and solving for temperature, one can approximate the boiling point of the substance.

The normal boiling point is a specific case. It's defined as the temperature at which the vapor pressure of the liquid equals standard atmospheric pressure (1 atm). Knowing the Antoine coefficients for a substance allows for the straightforward estimation of its normal boiling point. This provides a crucial reference point for understanding its volatility and behavior at different temperatures.

Accuracy Considerations

While the Antoine equation offers a user-friendly approach to vapor pressure estimation, it's important to acknowledge its accuracy limitations. As an empirical equation, its accuracy is inherently tied to the quality and range of the experimental data used to determine the Antoine coefficients.

Extrapolating the Antoine equation beyond its validated temperature range can lead to significant errors. The equation also assumes ideal behavior and doesn't account for complex intermolecular interactions. This can reduce accuracy, especially for substances that exhibit strong deviations from ideality.

For higher accuracy over wider temperature ranges, particularly near the critical point, more sophisticated vapor pressure models are available. The Wagner equation, for example, is a more complex equation that offers improved accuracy but requires more parameters and computational effort. Careful consideration should be given to the selection of the appropriate model based on the desired accuracy and the available data for the substance of interest.

Practical Applications and Resources: Tools for Vapor Pressure Estimation

Theoretical Underpinnings: Connecting to Thermodynamics The Antoine equation stands as a practical tool for estimating vapor pressure, a crucial property in thermodynamics and chemical engineering. This empirical equation provides a relatively simple yet effective method for approximating the vapor pressure of pure substances as a function of temperature. Building upon this foundation, let's explore the practical applications of the Antoine equation and the resources available to facilitate its use, ranging from coefficient databases to sophisticated measurement techniques.

Databases of Antoine Coefficients: The Cornerstone of Accurate Estimation

The accuracy of the Antoine equation hinges on the reliability of the A, B, and C coefficients.

These coefficients are substance-specific and are determined experimentally.

Fortunately, a wealth of data exists in various databases and software-integrated resources.

These databases provide readily accessible Antoine coefficients for a wide array of chemical compounds.

Examples of such resources include:

• NIST Chemistry WebBook: A comprehensive online resource offering thermochemical data, including Antoine coefficients for numerous substances.
• DIPPR Database: (Design Institute for Physical Properties Research) A subscription-based database widely used in the chemical engineering community.
• Perry's Chemical Engineers' Handbook: A standard reference containing tables of Antoine coefficients for common chemicals.

It is crucial to exercise caution when selecting coefficients, as values can vary between sources due to differences in experimental methods and data fitting procedures.

Always prioritize using validated and reputable data sources to ensure the accuracy of your vapor pressure estimations.

Computational Tools: From Simple Calculators to Advanced Simulations

The computational landscape offers a range of tools for leveraging the Antoine equation, catering to diverse needs and levels of complexity.

Online Calculators: Quick and Convenient Estimations

Numerous online calculators are readily available for quick vapor pressure estimations using the Antoine equation.

These calculators typically require the user to input the substance, temperature, and corresponding coefficients.

While convenient, it is important to verify the accuracy and reliability of these calculators, as the underlying data and calculation methods may not always be transparent.

Spreadsheets: Implementing the Antoine Equation for Custom Analysis

Spreadsheet software such as Microsoft Excel or Google Sheets provides a flexible platform for implementing the Antoine equation and performing custom calculations.

Users can input the coefficients and temperature values to generate vapor pressure curves and analyze the relationship between temperature and vapor pressure.

Spreadsheets also allow for incorporating additional data, such as experimental measurements, to compare and validate the estimations obtained from the Antoine equation.

Curve Fitting Software: Determining Coefficients from Experimental Data

When experimental vapor pressure data is available, curve fitting software can be used to determine the Antoine coefficients that best fit the data.

Software packages like OriginLab, MATLAB, or Python libraries such as SciPy offer powerful tools for performing nonlinear regression analysis and optimizing the coefficients to minimize the difference between the predicted and measured vapor pressures.

This approach is particularly useful when reliable coefficients are not available in existing databases or when dealing with complex mixtures.

Process Simulation Software: Integrating Vapor Pressure Models in Complex Systems

Process simulation software, such as Aspen Plus, CHEMCAD, or gPROMS, utilizes thermodynamic models, including the Antoine equation, to simulate and analyze chemical processes.

These software packages allow engineers to model complex systems involving multiple components, reactions, and unit operations.

The Antoine equation serves as one component of a more comprehensive thermodynamic framework, enabling accurate predictions of phase equilibria, vapor-liquid separations, and other process-related phenomena.

Measurement Tools: Experimental Determination of Vapor Pressure

While the Antoine equation provides a valuable estimation tool, experimental measurements are often necessary to validate the equation's accuracy or to determine vapor pressures for substances lacking reliable coefficient data.

Various techniques and instrumentation are employed for experimental vapor pressure determination:

• Static Methods: These methods involve measuring the pressure exerted by a substance in a closed system at a specific temperature. Manometers or pressure transducers are used to accurately measure the pressure.
• Dynamic Methods: These methods involve boiling the liquid and measuring the temperature at which the vapor pressure equals the external pressure. Ebulliometers are commonly used for this purpose.
• Gas Saturation Methods: These methods involve passing a carrier gas through the liquid and measuring the amount of vapor carried by the gas. This allows for calculation of the vapor pressure.
• Torsion or Quartz Crystal Microbalance (QCM) Methods: These methods can measure extremely low vapor pressures by measuring the mass of vapor adsorbed onto a surface.

The choice of method depends on the desired accuracy, the temperature range, and the nature of the substance being studied.

Analysis and Evaluation: Assessing the Accuracy and Limitations

The Antoine equation stands as a practical tool for estimating vapor pressure, a crucial property in thermodynamics and chemical engineering. This empirical equation provides a relatively simple yet effective method for approximating vapor pressure. However, its accuracy is not absolute, and understanding its limitations is paramount for its appropriate application. This section delves into the critical evaluation of the Antoine equation, providing insights into assessing its accuracy, understanding correlation, and recognizing situations where its application may not be suitable.

Understanding Correlation and Goodness of Fit

The utility of the Antoine equation rests on its ability to accurately represent the relationship between temperature and vapor pressure for a given substance. Assessing how well the equation fits experimental data is a critical step in validating its use. This involves understanding the concept of correlation, which describes the degree to which two variables are related.

A strong correlation indicates that changes in temperature are closely associated with predictable changes in vapor pressure, as described by the Antoine equation. Conversely, a weak correlation suggests a poor fit, potentially rendering the equation unreliable for that specific substance or temperature range.

Statistical Measures of Correlation

Several statistical measures can quantify the goodness of fit. R-squared (R²), also known as the coefficient of determination, is a widely used metric. It represents the proportion of the variance in the dependent variable (vapor pressure) that is predictable from the independent variable (temperature).

An R² value close to 1 indicates a strong correlation, suggesting that the Antoine equation accurately captures the relationship between temperature and vapor pressure. Values closer to 0, however, imply a poor fit.

It is important to note that R² alone is not a definitive measure. Visual inspection of the data and residuals (the differences between the predicted and observed values) is also crucial.

Residual plots can reveal systematic deviations or patterns that might not be evident from the R² value alone, indicating potential issues with the equation's applicability.

Limitations of the Antoine Equation

Despite its widespread use, the Antoine equation is subject to several limitations that must be carefully considered. These limitations arise from its empirical nature and simplifying assumptions.

Valid Temperature Ranges

One of the most significant limitations is the restricted temperature range for which the Antoine equation is valid. The constants (A, B, and C) are determined based on experimental data within a specific temperature interval.

Extrapolating the equation beyond this range can lead to significant errors and inaccurate vapor pressure estimations.

It is crucial to always verify the specified temperature range for the Antoine coefficients being used and to avoid applying the equation outside of this range. Many databases provide this range alongside the coefficient values.

Applicability to Non-Ideal Substances

The Antoine equation is most reliable for substances that exhibit relatively ideal behavior. For highly non-ideal substances, especially those with strong intermolecular interactions (e.g., hydrogen bonding), the equation's accuracy may be compromised. In such cases, more sophisticated vapor pressure models may be necessary.

Proximity to the Critical Point

The Antoine equation tends to lose accuracy as the temperature approaches the critical point of a substance. Near the critical point, the distinction between liquid and vapor phases becomes blurred, and the assumptions underlying the Antoine equation break down. Again, more complex equations of state are better suited for modeling vapor pressure in this region.

In summary, while the Antoine equation offers a convenient and practical means of estimating vapor pressure, a thorough understanding of its limitations is crucial for ensuring the reliability of the results. Careful consideration of the temperature range, substance ideality, and proximity to the critical point will guide users in making informed decisions about the applicability of this valuable tool.

Video: Antoine Equation: Vapor Pressure Made Easy

Play Video

So, next time you're wrestling with vapor pressure calculations, remember the Antoine equation! It might look a little intimidating at first, but with a little practice, you'll be predicting boiling points like a pro. Happy calculating!