Using Pythagorean Theorem: Real Life Examples

Using the Pythagorean Theorem, carpenters calculate precise diagonal measurements for staircases, ensuring each step aligns perfectly with the structure; right-angled triangles, a fundamental concept, form the basis of these calculations. Civil engineers apply the Pythagorean Theorem to design stable bridges, using this mathematical principle to distribute weight evenly across support beams, thus enhancing structural integrity. Navigation systems use this theorem to determine the shortest distance between two points on Earth, where GPS technology relies on geometric calculations to provide accurate location data. Architects frequently use the Pythagorean Theorem to design buildings, calculating roof slopes and corner angles to create aesthetically pleasing and structurally sound designs.

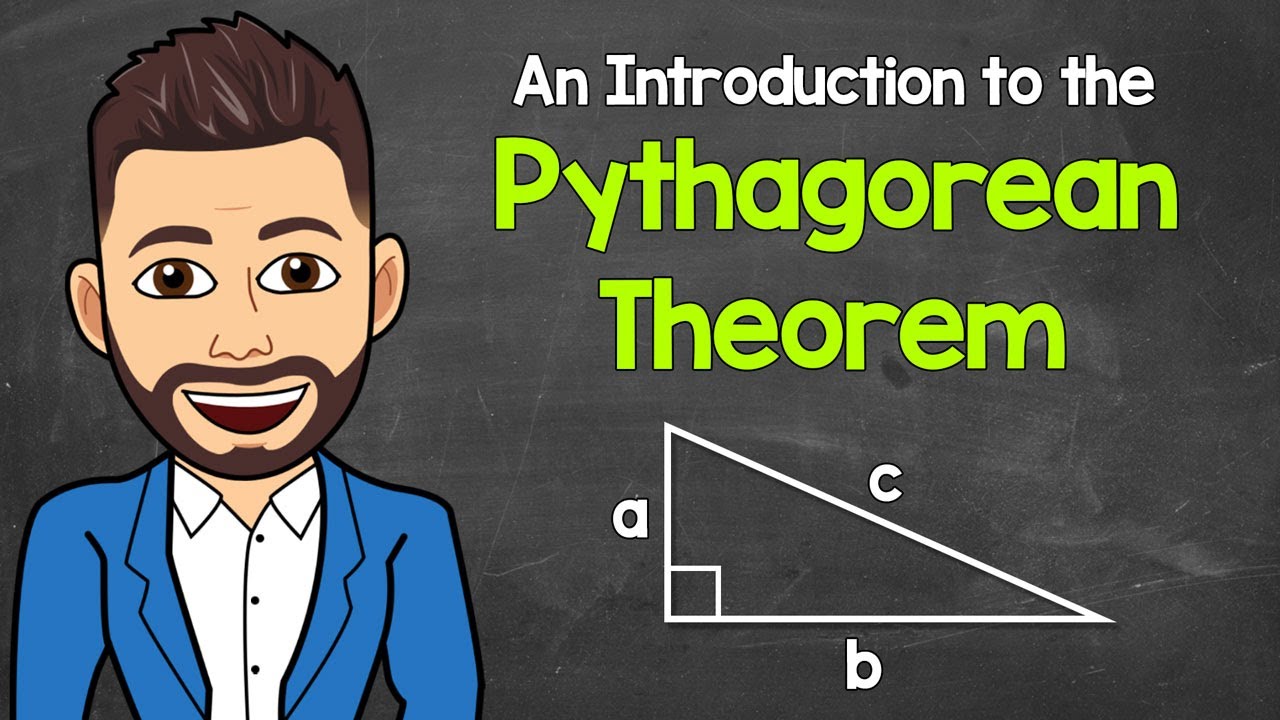
Image taken from the YouTube channel Math with Mr. J , from the video titled An Introduction to the Pythagorean Theorem | Math with Mr. J .

Still Relevant Today: Unveiling the Enduring Power of the Pythagorean Theorem

Ever watch a construction crew meticulously laying the foundation for a new building? Or seen a surveyor plotting out property lines with laser precision? What if I told you that behind these feats of engineering and measurement lies a mathematical principle as old as civilization itself?

We’re talking about the Pythagorean Theorem, of course!

This isn't just some dusty formula from your high school geometry textbook. It's a vibrant, living tool that continues to shape the world around us.

But why is it so important? Why does it persist? And how does this seemingly simple equation – a² + b² = c² – bridge the gap between abstract mathematics and the tangible reality of everyday life?

The Heart of the Matter: a² + b² = c²

At its core, the Pythagorean Theorem describes a fundamental relationship within a right triangle.

You know, that triangle with one angle measuring exactly 90 degrees.

It elegantly states that the sum of the squares of the two shorter sides (the legs, a and b) is equal to the square of the longest side (the hypotenuse, c). Simple, right?

Yet, it unlocks a universe of possibilities.

Bridging Math and Reality

The Pythagorean Theorem isn't just about triangles on paper. It's a fundamental principle linking abstract mathematics to practical applications across various fields.

It's the bedrock for calculating distances, ensuring structural integrity, and navigating the world around us. It allows us to measure with precision and build with confidence.

From builders squaring foundations to surveyors mapping landscapes, the theorem provides an indispensable framework for understanding and manipulating spatial relationships.

A Journey Through the Theorem

In this exploration, we’ll delve into the heart of this theorem, unpacking its components and understanding its geometric significance.

We'll journey through the real-world scenarios where it shines. Uncovering its practical uses in construction, surveying, navigation, and beyond.

Finally, we'll touch upon its mathematical connections, highlighting its place within the broader landscape of mathematical knowledge.

Prepare to rediscover the enduring power of Pythagoras. Get ready to see the world through a new, geometrically informed lens.

Understanding the Theorem: Decoding a² + b² = c²

Remember that foundation we were just talking about? Or those property lines? All of that relies on truly understanding the nuts and bolts, the ABCs if you will, of the Pythagorean Theorem. Let's break it down piece by piece so that we’re all on the same page about what this equation actually means.

Defining the Right Triangle: The Foundation of the Theorem

The Pythagorean Theorem only works with right triangles.

So, what defines a right triangle?

The 90-Degree Angle: Its Defining Characteristic

The most important feature is that it has one angle that is exactly 90 degrees. This is often marked with a small square in the corner of the triangle. That little square is your visual cue that you're dealing with a right triangle, and therefore, the Pythagorean Theorem can be applied.

The Hypotenuse: The Longest Side

The hypotenuse is the longest side of the right triangle, and it's always opposite the 90-degree angle. It's crucial to identify the hypotenuse correctly because it's a key piece in the equation. Think of it as the "star" of the triangle.

The Legs: The Sides Forming the Right Angle

The two shorter sides that form the 90-degree angle are called the legs. They are often referred to as sides a and b in the Pythagorean Theorem equation. Knowing which sides are the legs and which is the hypotenuse is half the battle!

Understanding the Equation: a² + b² = c²

Now that we know the parts of the right triangle, let’s dive into the equation itself. a² + b² = c²

a and b as Leg Lengths

In the equation, a and b represent the lengths of the two legs of the right triangle. You can choose either leg to be a or b; the order doesn't matter. What does matter is that you correctly identify the legs!

c as the Hypotenuse Length

c always represents the length of the hypotenuse. This is non-negotiable! It's crucial that c is always the hypotenuse length in order for the theorem to work.

Geometric Interpretation of Squaring: Area of Squares

The "squaring" part (a², b², c²) is also key to understanding the theorem. a² means a times a, and geometrically, it represents the area of a square with side length a.

The same applies to b² and c². Imagine drawing a square on each side of the right triangle. The area of the square on leg a plus the area of the square on leg b will always equal the area of the square on the hypotenuse (c).

That's the essence of the Pythagorean Theorem!

Pythagoras of Samos: The Man Behind the Math

Lastly, let's give credit where credit is due.

The theorem is named after the ancient Greek mathematician Pythagoras of Samos (although evidence suggests the concept was known before his time). Pythagoras founded a school that studied mathematics, philosophy, and music. While the theorem bears his name, it's a testament to the enduring power of mathematical discovery and its impact on our understanding of the world.

Real-World Applications: Where the Theorem Shines

Okay, enough theory! Let's talk about where the rubber meets the road. The Pythagorean Theorem isn't just some abstract concept confined to textbooks. It's a powerful tool used every single day by professionals across a surprising number of fields. From the blueprint of your house to the GPS guiding your car, this theorem is quietly working behind the scenes.

Let’s dive into specific examples and see how a² + b² = c² truly shines!

Construction: Building on a Solid Foundation

Construction is absolutely teeming with applications of the Pythagorean Theorem. We're talking builders, construction workers, architects – they're all constantly relying on it, whether they realize it or not.

Think about it: construction sites are basically giant puzzles, and the theorem helps ensure everything fits together perfectly, at precise right angles.

The 3-4-5 Triangle Trick: A Time-Tested Technique

One of the coolest (and oldest!) tricks in the book is the 3-4-5 triangle. It's a super simple way to create a perfect right angle using the theorem.

Measure 3 units along one side, 4 units along the other, and if the distance between the ends is exactly 5 units, you've got yourself a 90-degree angle.

This is especially useful for squaring up foundations, walls, and corners. No fancy equipment needed!

Construction Companies and the Power of Precision

Larger construction companies use more sophisticated tools, of course. But the underlying principle is always the Pythagorean Theorem.

They use it for everything from calculating roof slopes to determining the diagonal lengths of structures. It all needs to be perfect!

Without this theorem, buildings would be unstable and unsafe. That means no skyscrapers.

Land Surveying: Mapping the World with Accuracy

Surveyors are the unsung heroes of land measurement, and they're completely reliant on the Pythagorean Theorem.

They use it to calculate areas, determine property lines, and establish accurate distances across all kinds of terrain.

Imagine trying to map out a large piece of land without knowing how to calculate the distance between two points that aren't directly in line with each other. Impossible!

The Pythagorean Theorem provides the mathematical backbone for creating accurate maps and property records. It's the foundation of modern surveying.

Navigation: Charting a Course

Navigation, especially in its historical context, relies heavily on the Pythagorean Theorem.

Calculating distances and headings, especially at sea, used to require a deep understanding of geometry.

Even with modern GPS systems, the underlying calculations often involve the theorem for determining precise positioning.

Without it, early explorers would have been even more lost than they sometimes were!

Distance Calculation: Beyond Triangles

The applications extend far beyond just right triangles. Anytime you need to calculate the distance between two points, and you can create an imaginary right triangle with those points, you can use the theorem.

This pops up in video game design (calculating distances between characters), robotics (planning robot movements), and even in graphic design (determining the length of a line drawn diagonally across a screen).

It’s a versatile tool that keeps on giving.

Tools of the Trade: Applying the Theorem Practically

Okay, enough theory! Let's talk about where the rubber meets the road. The Pythagorean Theorem isn't just some abstract concept confined to textbooks. It's a powerful tool used every single day by professionals across a surprising number of fields. From the blueprint of your house to the GPS guiding your car, this theorem is quietly working behind the scenes. But how do people actually use it? Let's dive into the tools that bring this mathematical marvel to life, from the simplest instruments to the most sophisticated tech.

The Unsung Heroes: Low-Tech Tools

Before we had fancy gadgets and apps, people relied on ingenuity and simple instruments. These "low-tech" tools are still incredibly relevant today. They provide a tangible connection to the theorem. They can also be valuable for quick checks or situations where technology might fail us.

Rulers and Tape Measures: Measuring with Precision

At its core, the Pythagorean Theorem deals with the lengths of the sides of a right triangle. Accurate measurement is therefore paramount. Rulers and tape measures, in both imperial and metric units, are essential for determining the values of a, b, and c.

Think about it: a slight inaccuracy in measuring just one side can throw off the entire calculation. Whether it's a carpenter measuring wood for a frame or a landscaper planning a garden, precision is key.

Squares: Ensuring Right Angles

The Pythagorean Theorem only applies to right triangles. So, ensuring that you actually have a right triangle is crucial. That's where squares come in. These tools, typically made of metal or plastic, guarantee a perfect 90-degree angle.

From the humble try square to more elaborate speed squares, these tools are indispensable for verifying that the sides of a structure or design are truly perpendicular. They provide the foundation for the entire Pythagorean calculation. Without a perfect right angle, the theorem simply doesn't hold.

Modern Marvels: Calculators and Beyond

While rulers and squares provide the foundation, modern technology supercharges the application of the Pythagorean Theorem. Calculators and specialized software take the drudgery out of the calculations. They allow professionals to focus on design, problem-solving, and ensuring accuracy.

The Ubiquitous Calculator

Let's face it: calculating square roots by hand can be tedious. Especially when dealing with complex measurements. The calculator, from the basic scientific model to specialized construction calculators, automates these calculations. It dramatically reduces the chance of human error.

It quickly performs square root operations and makes complex calculations straightforward. This frees up valuable time and mental energy. The calculator makes it easier to apply the theorem in real-time.

Software Solutions: CAD and More

Beyond basic calculations, specialized software like CAD (Computer-Aided Design) programs integrate the Pythagorean Theorem seamlessly into design and planning processes. These programs allow architects and engineers to accurately model structures, calculate distances, and verify angles with incredible precision.

The theorem becomes an invisible but essential component. It ensures structural integrity and accurate spatial relationships within the design. This is essential for bridges and buildings.

Mathematical Connections: Beyond the Basics

Okay, so we've seen the Pythagorean Theorem in action, building houses and mapping land. But this isn't just a handy trick for construction workers and surveyors. It's a gateway to deeper mathematical concepts. Let's delve into some fascinating connections that elevate a² + b² = c² from a simple equation to a cornerstone of mathematical thought.

Pythagorean Triples: A Family of Numbers

Ever stumble upon a set of whole numbers that perfectly satisfy the Pythagorean Theorem? Those are Pythagorean Triples!

They're sets of three positive integers, a, b, and c, such that a² + b² = c².

The most famous example? That's the 3-4-5 triangle. I'm sure you've seen it before! It's a classic. Because 3² + 4² = 5² (9 + 16 = 25), these numbers form a Pythagorean Triple.

But it doesn't stop there. Other common examples include 5-12-13 and 8-15-17.

These aren't just random sets of numbers.

They follow patterns and have been studied for centuries.

Why are they useful? Because they offer whole number solutions, simplifying calculations in fields like carpentry. Instead of working with decimals, you can rely on whole numbers to quickly build right triangles.

That's great for efficiency, accuracy, and fewer headaches!

The Square Root Connection

Here's where things get a little more abstract – but stick with me! The Pythagorean Theorem is intrinsically linked to the concept of square roots. Remember that a² represents the area of a square with side a.

So, when we solve for the hypotenuse, c, we're essentially finding the square root of (a² + b²).

This means that the length of the hypotenuse is the side length of a square whose area is the sum of the areas of the squares formed by the two legs.

Woah, right?

This connection highlights the relationship between geometry (squares, triangles) and algebra (square roots, equations).

It's a beautiful illustration of how different mathematical concepts intertwine! And, this is precisely why this equation is so important!

Euclid's Elegant Proof in Elements

Ready for a journey back in time? Around 300 BC, Euclid, the "father of geometry," included a proof of the Pythagorean Theorem in his monumental work, Elements.

Euclid's proof isn't just any proof; it's a visual masterpiece.

He uses geometric constructions to demonstrate that the area of the square built upon the hypotenuse is equal to the sum of the areas of the squares built upon the legs.

The proof involves dissecting the larger squares and rearranging them to perfectly fit over the square on the hypotenuse.

It's an incredibly elegant and intuitive demonstration of the theorem's validity. It also is an undeniable visual confirmation.

And it's also a powerful reminder that mathematical truths can be revealed through visual reasoning. If you ever get a chance to see a diagram of Euclid's proof, definitely check it out.

It's a classic for a reason!

Video: Using Pythagorean Theorem: Real Life Examples

Play Video

So, next time you're wondering if that shelf will fit, or trying to figure out the quickest route across a park, remember old Pythagoras. Turns out, knowing a² + b² = c² can be surprisingly useful in everyday life! Give using the Pythagorean Theorem a try – you might be surprised how often it comes in handy.