Mastering Percent Dissociation: A Complete Guide
Percent dissociation, a pivotal concept in chemistry, directly influences our understanding of equilibrium. Acid-base chemistry, one critical area where it finds application, often utilizes percent dissociation to quantify the strength of weak acids and bases. The Henderson-Hasselbalch equation provides a mathematical framework for relating percent dissociation to pH and pKa, enabling precise calculations. Furthermore, the National Institute of Standards and Technology (NIST) maintains databases of thermodynamic properties that are invaluable when assessing percent dissociation under varying conditions.
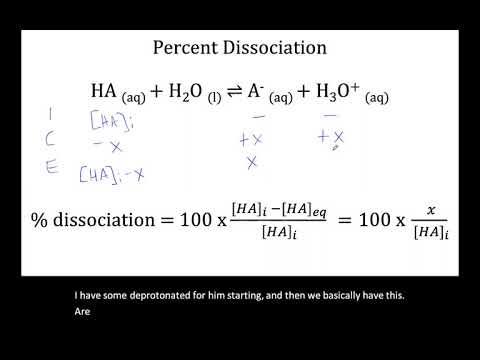
Image taken from the YouTube channel CSB SJU Chemistry , from the video titled Percent Dissociation .
Percent dissociation is a fundamental concept in chemistry, particularly within the realms of acid-base chemistry and chemical equilibrium. It offers a quantitative measure of the extent to which a substance, typically a weak acid or base, breaks apart into its constituent ions when dissolved in a solution. This seemingly simple metric holds profound implications for understanding and predicting chemical behavior in various systems.
What is Percent Dissociation? Definition and Significance
Percent dissociation, often represented as α%, quantifies the proportion of an original solute that has dissociated into ions in a solution. Mathematically, it is expressed as:
α% = (Concentration of dissociated species / Initial concentration of solute) * 100
The initial concentration of the solute refers to the concentration of the acid or base before any dissociation occurs. The concentration of dissociated species refers to the concentration of the acid or base after it has reached equilibrium.
For instance, a 5% dissociation for a weak acid means that only 5% of the original acid molecules have broken down into ions, while the remaining 95% remain undissociated.
The significance of percent dissociation lies in its ability to characterize the strength of weak acids and bases. Unlike strong acids and bases, which dissociate almost completely in solution, weak acids and bases only dissociate partially. Percent dissociation provides a direct measure of this partial dissociation, allowing for comparisons between different weak acids and bases.
Why is Percent Dissociation Important?
Percent dissociation is a crucial parameter for several reasons:
-
Predicting Solution Behavior: It helps predict the concentration of ions in solution, which directly influences properties like pH, conductivity, and reactivity.
-
Understanding Chemical Equilibrium: It provides insight into the position of the equilibrium between the undissociated solute and its ions. A higher percent dissociation indicates that the equilibrium favors the formation of ions.
-
Applications Across Disciplines: Its importance extends beyond the laboratory, playing a vital role in fields like environmental science (understanding the impact of acid rain), pharmaceutical science (predicting drug bioavailability), and industrial chemistry (optimizing reaction conditions).
Overview of Factors Influencing Percent Dissociation
Several factors can influence the percent dissociation of a weak acid or base. Understanding these factors is crucial for controlling and predicting chemical behavior:
-
Concentration: Generally, as the concentration of a weak acid or base decreases (dilution), the percent dissociation increases. This is because, according to Le Chatelier's principle, diluting the solution shifts the equilibrium towards the side with more ions to counteract the change in concentration.
-
Temperature: Temperature affects the equilibrium constant (Ka or Kb) of the dissociation reaction. Since Ka and Kb are directly related to the extent of dissociation, changing the temperature will alter the percent dissociation. For endothermic dissociation reactions, increasing the temperature will increase percent dissociation, while the opposite is true for exothermic reactions.
-
Common Ion Effect: The presence of a common ion (an ion already present in the solution that is also a product of the weak acid or base dissociation) decreases the percent dissociation. This is another application of Le Chatelier's principle; the presence of the common ion shifts the equilibrium towards the undissociated form of the acid or base.
Percent dissociation reveals how much of an acid or base turns into ions when dissolved. However, before diving deeper into percent dissociation, it’s essential to solidify the fundamental concepts upon which it's built. Understanding the nature of acids and bases, the crucial role of water in their interactions, and the principles of chemical equilibrium are all foundational to grasping the meaning and implications of percent dissociation.
Fundamentals: Acids, Bases, and Chemical Equilibrium
To truly understand percent dissociation, a firm grasp of acids, bases, and chemical equilibrium is essential. These concepts provide the framework for understanding how substances behave in solution and how their interactions drive chemical processes.
Understanding Acids and Bases: A Multifaceted Definition
The definitions of acids and bases have evolved over time, with each new definition building upon previous ones to provide a more comprehensive understanding. Here's a quick look at three important definitions:
-
Arrhenius Definition: This is the oldest and simplest definition. It defines acids as substances that produce hydrogen ions (H+) in aqueous solution, and bases as substances that produce hydroxide ions (OH-) in aqueous solution.
-
Bronsted-Lowry Definition: This definition expands upon the Arrhenius definition. It defines acids as proton (H+) donors and bases as proton acceptors. This definition is broader than the Arrhenius definition because it does not limit acids and bases to aqueous solutions.
-
Lewis Definition: This is the most general definition. It defines acids as electron pair acceptors and bases as electron pair donors. This definition encompasses all Bronsted-Lowry acids and bases, as well as many other substances that do not contain hydrogen ions or hydroxide ions.
The Role of Water in Acid-Base Chemistry
Water plays a critical role in acid-base chemistry. It acts as both an acid and a base, a property known as amphoterism.
Water can accept a proton from an acid, acting as a Bronsted-Lowry base. Water can also donate a proton to a base, acting as a Bronsted-Lowry acid.
This dual nature allows water to participate in acid-base reactions and to influence the equilibrium of these reactions.
The autoionization of water, where water molecules react with each other to form hydronium ions (H3O+) and hydroxide ions (OH-), is a prime example of its amphoteric behavior.
The concentration of these ions determines the acidity or basicity of a solution.
Equilibrium Constant (Ka, Kb): Definition and Importance
Chemical reactions, especially acid-base reactions involving weak acids or bases, often reach a state of equilibrium.
At equilibrium, the rates of the forward and reverse reactions are equal, and the concentrations of reactants and products remain constant.
The equilibrium constant (K) is a value that expresses the ratio of products to reactants at equilibrium. For acid-base reactions, we specifically use Ka and Kb.
-
Ka (Acid Dissociation Constant): This constant quantifies the strength of a weak acid. It represents the equilibrium constant for the dissociation of an acid into its conjugate base and a proton. A higher Ka value indicates a stronger acid.
-
Kb (Base Dissociation Constant): This constant quantifies the strength of a weak base. It represents the equilibrium constant for the dissociation of a base into its conjugate acid and a hydroxide ion. A higher Kb value indicates a stronger base.
These constants are essential for predicting the extent to which an acid or base will dissociate in solution, and therefore, are critical to determining the pH of the solution.
Dissociation Constant: Relationship Between Ka, Kb, and Strength of Acids and Bases
The dissociation constants, Ka and Kb, are directly related to the strength of acids and bases.
A strong acid will have a large Ka value, indicating that it readily dissociates in solution. Conversely, a weak acid will have a small Ka value, indicating that it only partially dissociates.
Similarly, a strong base will have a large Kb value, while a weak base will have a small Kb value.
For conjugate acid-base pairs, Ka and Kb are related by the following equation:
Kw = Ka * Kb
Where Kw is the ion product of water (1.0 x 10-14 at 25°C). This relationship highlights the inverse relationship between the strength of an acid and its conjugate base. A strong acid will have a weak conjugate base, and vice versa. Understanding these fundamental relationships between Ka, Kb, and the strength of acids and bases is crucial for predicting the behavior of these substances in solution.
Percent dissociation reveals how much of an acid or base turns into ions when dissolved. However, before diving deeper into percent dissociation, it’s essential to solidify the fundamental concepts upon which it's built. Understanding the nature of acids and bases, the crucial role of water in their interactions, and the principles of chemical equilibrium are all foundational to grasping the meaning and implications of percent dissociation.
Now, equipped with a strong foundation in acid-base chemistry and equilibrium principles, we can explore a powerful tool for quantifying the extent to which weak acids and bases dissociate in solution. This tool is the ICE table method, which provides a systematic approach to calculating percent dissociation.
Calculating Percent Dissociation: The ICE Table Method
The ICE table method is a cornerstone of chemical equilibrium calculations, especially when dealing with weak acids and bases. It allows us to determine the equilibrium concentrations of reactants and products, which are essential for calculating percent dissociation.
The ICE Table Method: A Step-by-Step Guide
ICE stands for Initial, Change, and Equilibrium. The ICE table is a structured way to organize the information needed to solve equilibrium problems. Here's a breakdown of the steps involved:
-
Write the Balanced Chemical Equation: Start by writing the balanced equation for the dissociation of the weak acid or base in water. This equation is crucial for determining the stoichiometry of the reaction.
-
Set up the ICE Table: Create a table with three rows (Initial, Change, Equilibrium) and columns for each reactant and product in the balanced equation.
-
Fill in the Initial Concentrations (I): Determine the initial concentrations of all species involved. Typically, the initial concentration of the weak acid or base is given, and the initial concentrations of the products (H+ or OH-) are assumed to be zero (before dissociation).
-
Determine the Change in Concentrations (C): Use the stoichiometry of the balanced equation to express the change in concentration of each species in terms of a single variable, usually "x". For example, if one mole of acid dissociates to produce one mole of H+ and one mole of A-, the change in concentration of the acid will be -x, and the change in concentration of H+ and A- will be +x.
-
Calculate the Equilibrium Concentrations (E): Add the change in concentration to the initial concentration for each species to obtain the equilibrium concentration. These concentrations will be expressed in terms of "x".
-
Write the Equilibrium Constant Expression: Write the expression for the equilibrium constant (Ka for weak acids, Kb for weak bases) in terms of the equilibrium concentrations.
-
Solve for "x": Substitute the equilibrium concentrations (in terms of "x") into the equilibrium constant expression and solve for "x". This may involve using the quadratic formula or making simplifying approximations (discussed later).
-
Calculate Percent Dissociation: Once you have found the value of "x", calculate the percent dissociation using the following formula:
Percent Dissociation = ([H+] or [OH-] at equilibrium / Initial concentration of acid or base) 100%
**
Calculating Percent Dissociation for Weak Acids
For a weak acid (HA) dissociating in water:
HA(aq) + H2O(l) ⇌ H3O+(aq) + A-(aq)
The ICE table would look something like this:
| HA | H3O+ | A- | |
|---|---|---|---|
| Initial (I) | [HA]₀ | 0 | 0 |
| Change (C) | -x | +x | +x |
| Equilibrium (E) | [HA]₀-x | x | x |
Ka = [H3O+][A-] / [HA] = x² / ([HA]₀ - x)
Calculating Percent Dissociation for Weak Bases
For a weak base (B) reacting with water:
B(aq) + H2O(l) ⇌ BH+(aq) + OH-(aq)
The ICE table would look something like this:
| B | BH+ | OH- | |
|---|---|---|---|
| Initial (I) | [B]₀ | 0 | 0 |
| Change (C) | -x | +x | +x |
| Equilibrium (E) | [B]₀-x | x | x |
Kb = [BH+][OH-] / [B] = x² / ([B]₀ - x)
Example Problems: Walkthroughs with Detailed Explanations
Let's solidify this with a couple of examples.
Example 1: Weak Acid
Calculate the percent dissociation of a 0.10 M solution of acetic acid (CH3COOH), given that its Ka is 1.8 x 10⁻⁵.
-
Balanced Equation: CH3COOH(aq) ⇌ H+(aq) + CH3COO-(aq)
-
ICE Table:
CH3COOH H+ CH3COO- Initial (I) 0.10 0 0 Change (C) -x +x +x Equilibrium (E) 0.10-x x x -
Ka Expression: Ka = [H+][CH3COO-] / [CH3COOH] = x² / (0.10 - x)
-
Solve for x: 1.8 x 10⁻⁵ = x² / (0.10 - x). Assuming x is small compared to 0.10, we can approximate 0.10 - x ≈ 0.10.
Therefore, 1.8 x 10⁻⁵ = x² / 0.10
x² = 1.8 x 10⁻⁶
x = √(1.8 x 10⁻⁶) = 1.34 x 10⁻³ M
-
Percent Dissociation: (1.34 x 10⁻³ / 0.10)** 100% = 1.34%
Example 2: Weak Base
Calculate the percent dissociation of a 0.050 M solution of ammonia (NH3), given that its Kb is 1.8 x 10⁻⁵.
-
Balanced Equation: NH3(aq) + H2O(l) ⇌ NH4+(aq) + OH-(aq)
-
ICE Table:
NH3 NH4+ OH- Initial (I) 0.050 0 0 Change (C) -x +x +x Equilibrium (E) 0.050-x x x -
Kb Expression: Kb = [NH4+][OH-] / [NH3] = x² / (0.050 - x)
-
Solve for x: 1.8 x 10⁻⁵ = x² / (0.050 - x). Assuming x is small compared to 0.050, we can approximate 0.050 - x ≈ 0.050.
Therefore, 1.8 x 10⁻⁵ = x² / 0.050
x² = 9.0 x 10⁻⁷
x = √(9.0 x 10⁻⁷) = 9.49 x 10⁻⁴ M
-
Percent Dissociation: (9.49 x 10⁻⁴ / 0.050) * 100% = 1.90%
Approximations and Assumptions: When are they valid?
In the previous examples, we made a simplifying assumption: that "x" is small compared to the initial concentration of the acid or base. This assumption is valid when the Ka or Kb value is small (typically less than 10⁻⁴) and the initial concentration is relatively high.
The 5% Rule:
A common rule of thumb is the "5% rule". If the percent dissociation is less than 5%, the approximation is considered valid. If the percent dissociation is greater than 5%, the approximation is not valid, and you must use the quadratic formula to solve for "x".
When the Approximation Fails:
If the Ka or Kb value is relatively large, or the initial concentration is very low, the approximation may not be valid. In these cases, you must use the quadratic formula to solve for "x":
ax² + bx + c = 0
x = (-b ± √(b² - 4ac)) / 2a
Using the quadratic formula provides a more accurate solution, but it also increases the complexity of the calculation. Therefore, it's crucial to always check the validity of the approximation before relying on it. If the approximation is invalid, the quadratic formula is the necessary alternative.
After becoming proficient with the ICE table method, we recognize that percent dissociation isn't a static property. Several factors in the chemical environment can shift the equilibrium, altering the degree to which an acid or base dissociates. Understanding these influences allows for a more nuanced prediction and control of chemical reactions.
Factors Influencing Percent Dissociation
Percent dissociation, the extent to which an acid or base dissociates into ions in solution, is not a fixed value. It is sensitive to changes in the surrounding environment. Understanding the factors that influence percent dissociation is crucial for controlling and predicting the behavior of weak acids and bases in various chemical and biological systems. Three primary factors govern the extent of dissociation: concentration, temperature, and the presence of common ions.
The Impact of Concentration: Dilution and its Effect on Percent Dissociation
The concentration of a weak acid or base plays a significant role in its percent dissociation. Generally, dilution increases the percent dissociation of weak acids and bases. This may seem counterintuitive at first, but it is a direct consequence of Le Chatelier's principle and the equilibrium expression.
When a solution is diluted, the concentrations of all species (including the ions formed by dissociation) decrease. According to Le Chatelier's principle, the equilibrium will shift to counteract this stress by favoring the side of the reaction that has more particles in solution.
For a weak acid or base, this means shifting towards further dissociation.
To illustrate, consider the dissociation of a weak acid, HA, in water:
HA(aq) + H₂O(l) ⇌ H₃O⁺(aq) + A⁻(aq)
Upon dilution, the system will try to restore the original concentration ratios by producing more H₃O⁺ and A⁻ ions, thus increasing the percent dissociation of HA.
However, it's important to note that while the percent dissociation increases with dilution, the absolute concentration of ions may decrease. The key takeaway is that the equilibrium shifts to favor dissociation, but the overall amount of acid or base present has decreased due to dilution.
The Role of Temperature
Temperature is another critical factor that influences percent dissociation. The effect of temperature depends on whether the dissociation reaction is endothermic or exothermic.
For endothermic reactions (those that absorb heat), increasing the temperature favors the forward reaction (dissociation). This leads to a higher percent dissociation because the equilibrium shifts to consume the added heat, producing more ions.
Conversely, for exothermic reactions (those that release heat), increasing the temperature favors the reverse reaction. This results in a lower percent dissociation as the equilibrium shifts to consume the added heat by reducing the amount of ions in the solution.
It's crucial to remember that the enthalpy change (ΔH) of the dissociation reaction determines the temperature dependence.
If ΔH is positive (endothermic), increasing temperature increases percent dissociation.
If ΔH is negative (exothermic), increasing temperature decreases percent dissociation.
In many cases, the dissociation of weak acids and bases is endothermic. However, it's essential to consult thermodynamic data or experimental observations to determine the specific temperature dependence for each substance.
Common Ion Effect and its Influence on Percent Dissociation
The common ion effect describes the decrease in the dissociation of a weak acid or base when a soluble salt containing a common ion is added to the solution. This effect is a direct application of Le Chatelier's principle.
Consider the dissociation of acetic acid (CH₃COOH) in water:
CH₃COOH(aq) + H₂O(l) ⇌ H₃O⁺(aq) + CH₃COO⁻(aq)
If we add sodium acetate (CH₃COONa) to this solution, sodium acetate will completely dissolve and provide a large amount of the common ion, acetate (CH₃COO⁻). This addition of acetate ions shifts the equilibrium to the left, suppressing the dissociation of acetic acid.
Consequently, the percent dissociation of acetic acid decreases in the presence of the common ion, acetate.
The common ion effect is widely used in controlling the pH of buffer solutions. By carefully selecting the concentrations of a weak acid and its conjugate base (or a weak base and its conjugate acid), one can create a buffer solution that resists changes in pH upon the addition of small amounts of acid or base. The common ion effect ensures that the concentrations of the weak acid and its conjugate base remain relatively constant, thereby stabilizing the pH.
After becoming proficient with the ICE table method, we recognize that percent dissociation isn't a static property. Several factors in the chemical environment can shift the equilibrium, altering the degree to which an acid or base dissociates. Understanding these influences allows for a more nuanced prediction and control of chemical reactions. This brings us to a crucial comparison: how does the behavior of strong acids and bases, which we often treat as fully dissociating, differ from that of their weaker counterparts when we consider percent dissociation?
Percent Dissociation: Strong vs. Weak Acids and Bases
The concepts of strong and weak acids and bases are fundamental in chemistry, but understanding their dissociation behavior requires a closer examination. The key difference lies in their extent of dissociation in solution, which directly impacts their percent dissociation values. Strong acids and bases undergo virtually complete dissociation, while weak acids and bases only partially dissociate, establishing an equilibrium between the undissociated species and its ions.
Strong Acids: Complete Dissociation and its Implications
Strong acids, such as hydrochloric acid (HCl), sulfuric acid (H₂SO₄), and nitric acid (HNO₃), are defined by their almost complete ionization in aqueous solutions.
This means that for a given concentration of a strong acid, the concentration of hydrogen ions (H⁺) is essentially equal to the initial concentration of the acid.
As a result, the percent dissociation of a strong acid is considered to be virtually 100%.
While technically, even strong acids might have a minuscule concentration of undissociated acid at equilibrium, this amount is generally negligible for most practical calculations.
This complete dissociation has significant implications:
-
Simplified Calculations: pH calculations for strong acid solutions are straightforward because the [H⁺] is approximately equal to the initial acid concentration.
-
High Reactivity: Solutions of strong acids are highly reactive due to the high concentration of H⁺ ions.
-
Corrosive Nature: The complete dissociation contributes to their corrosive nature.
Strong Bases: Complete Dissociation and its Implications
Similar to strong acids, strong bases, such as sodium hydroxide (NaOH) and potassium hydroxide (KOH), undergo nearly complete dissociation in aqueous solutions.
This results in a high concentration of hydroxide ions (OH⁻), with the percent dissociation being close to 100%.
Like strong acids, the assumption of complete dissociation simplifies calculations and explains their properties:
-
Simplified Calculations: pOH and pH calculations are simplified due to the near-complete dissociation.
-
Strong Electrolytes: Strong bases are strong electrolytes because they produce a high concentration of ions in solution, facilitating electrical conductivity.
-
Caustic Nature: The high concentration of OH⁻ ions makes them caustic and capable of causing severe burns.
Weak Acids and Weak Bases: Equilibrium and Percent Dissociation
Unlike their strong counterparts, weak acids and bases do not fully dissociate in water. Instead, they exist in equilibrium with their ions.
For example, acetic acid (CH₃COOH), a weak acid, dissociates according to the following equilibrium:
CH₃COOH(aq) ⇌ H⁺(aq) + CH₃COO⁻(aq)
The extent of this dissociation is quantified by the acid dissociation constant, Ka, which indicates the ratio of products to reactants at equilibrium.
A smaller Ka value signifies a lower degree of dissociation. Similarly, weak bases, like ammonia (NH₃), react with water to produce hydroxide ions (OH⁻) and their conjugate acids, establishing an equilibrium governed by the base dissociation constant, Kb.
Because the dissociation is incomplete, the percent dissociation for weak acids and bases is significantly less than 100%. The exact value depends on the Ka or Kb value and the initial concentration of the acid or base.
Comparing Percent Dissociation Values for Different Acids and Bases
The key takeaway is the stark contrast in percent dissociation values:
-
Strong Acids and Bases: Percent dissociation ≈ 100%
-
Weak Acids and Bases: Percent dissociation << 100% (and dependent on Ka/Kb and concentration)
This difference stems from the fundamental nature of their interaction with water. Strong acids and bases react virtually completely, while weak acids and bases reach an equilibrium state with a substantial amount of undissociated species present.
Furthermore, the percent dissociation of weak acids and bases is not a fixed value; it changes with concentration and temperature, as we've discussed. Strong acids and bases, however, maintain their near-complete dissociation regardless of concentration changes.
Understanding this distinction is vital for accurately predicting the behavior of acids and bases in various chemical and biological systems and for performing accurate calculations related to pH, buffering capacity, and titration.
After becoming proficient with the ICE table method, we recognize that percent dissociation isn't a static property. Several factors in the chemical environment can shift the equilibrium, altering the degree to which an acid or base dissociates. Understanding these influences allows for a more nuanced prediction and control of chemical reactions. This brings us to a crucial comparison: how does the behavior of strong acids and bases, which we often treat as fully dissociating, differ from that of their weaker counterparts when we consider percent dissociation?
pH, pKa, and Percent Dissociation: Interrelationships
The interplay between pH, pKa, and percent dissociation forms a cornerstone of acid-base chemistry. These concepts are not isolated; they are intimately linked, offering a comprehensive understanding of acid and base behavior in solution. By grasping these interrelationships, one can predict and control chemical reactions with greater precision.
Understanding pH and its Relationship to Acids and Bases
pH, a measure of the hydrogen ion concentration ([H+]) in a solution, dictates its acidity or basicity. The pH scale, ranging from 0 to 14, provides a quantitative way to express the concentration of H+ ions.
Acidic solutions have a pH less than 7, indicating a higher concentration of H+ ions. Conversely, basic, or alkaline, solutions have a pH greater than 7, indicating a lower concentration of H+ ions. A pH of 7 signifies a neutral solution, where the concentration of H+ ions equals the concentration of hydroxide ions (OH-).
The relationship between pH and [H+] is logarithmic, defined by the equation:
pH = -log[H+]
This logarithmic scale means that a change of one pH unit represents a tenfold change in [H+].
pKa: Definition and its Correlation with Acid Strength
pKa is a quantitative measure of acid strength. It represents the negative base-10 logarithm of the acid dissociation constant (Ka).
pKa = -log(Ka)
The Ka value reflects the extent to which an acid dissociates in solution. A larger Ka value indicates a stronger acid, meaning it dissociates to a greater extent, releasing more H+ ions into the solution.
The pKa provides a more convenient way to express acid strength. A lower pKa value corresponds to a stronger acid.
This inverse relationship is important to remember. Strong acids have small or even negative pKa values, while weak acids have larger pKa values.
The pKa is an intrinsic property of a specific acid molecule, independent of its concentration. It provides a reliable benchmark for comparing the relative strengths of different acids.
Using pKa Values to Predict Percent Dissociation
The pKa value is a powerful tool for predicting the percent dissociation of a weak acid. While the ICE table method provides a precise calculation, the pKa offers a quicker, more intuitive understanding.
The Henderson-Hasselbalch equation connects pH, pKa, and the ratio of conjugate base ([A-]) to undissociated acid ([HA]):
pH = pKa + log([A-]/[HA])
When the pH of a solution is equal to the pKa of the acid, the concentrations of the acid and its conjugate base are equal ([HA] = [A-]). This means that at pH = pKa, the acid is 50% dissociated.
If the pH is significantly lower than the pKa (more acidic conditions), the equilibrium shifts towards the undissociated acid ([HA] predominates). The percent dissociation will be less than 50%.
Conversely, if the pH is significantly higher than the pKa (more basic conditions), the equilibrium shifts towards the conjugate base ([A-] predominates). The percent dissociation will be greater than 50%.
By comparing the pH of the solution to the pKa of the acid, one can quickly estimate the approximate percent dissociation without performing detailed calculations. This estimation is especially useful in buffer systems, where the relative concentrations of acid and conjugate base are critical for maintaining a stable pH.
Calculating pH from Percent Dissociation
While pKa helps predict percent dissociation, the reverse is also true: knowing the percent dissociation allows one to calculate the pH of the solution.
For a weak acid, HA, that dissociates according to the equation:
HA(aq) ⇌ H+(aq) + A-(aq)
If we know the initial concentration of the acid ([HA]₀) and the percent dissociation, we can determine the equilibrium concentrations of H+ and A-.
For example, if a 0.1 M solution of a weak acid has a percent dissociation of 5%, then the concentration of H+ at equilibrium is 0.05 * 0.1 M = 0.005 M.
Using the definition of pH, we can then calculate the pH:
pH = -log[H+] = -log(0.005) ≈ 2.3
This calculation demonstrates how percent dissociation directly relates to the hydrogen ion concentration, and thus, the pH of the solution. Understanding this relationship is crucial for applications such as titrations, where monitoring the pH change can indicate the equivalence point.
Real-World Applications of Percent Dissociation
Having established the theoretical underpinnings of percent dissociation and its relationships with pH and pKa, it is essential to explore its practical significance. This concept transcends academic exercises, finding critical applications across diverse fields. From the intricacies of biological systems to the design of effective pharmaceuticals, understanding percent dissociation offers invaluable insights and drives innovation.
Buffer Solutions: The Linchpin of Stability
Buffer solutions are ubiquitous in both natural and industrial processes. Their ability to resist changes in pH upon the addition of acids or bases is fundamental to maintaining stable chemical environments.
Percent dissociation plays a crucial role in determining buffer capacity. This capacity refers to the amount of acid or base a buffer can neutralize before significant pH changes occur.
The effective buffering range is typically within one pH unit of the pKa value of the weak acid component. This is where the percent dissociation of the weak acid is most sensitive to changes in pH. A higher concentration of the buffer components generally leads to a greater buffer capacity.
Titration Curves: Decoding Chemical Reactions
Titration is an analytical technique used to determine the concentration of a substance. The titration curve, a plot of pH versus the volume of titrant added, provides a visual representation of the reaction's progress.
Percent dissociation is intrinsically linked to the shape of the titration curve, particularly at the equivalence point and the half-equivalence point.
At the half-equivalence point, where half of the weak acid has been neutralized, the pH is equal to the pKa of the acid. Here, the percent dissociation provides direct insight into the acid's strength and its behavior at that specific stage of the titration.
Environmental Chemistry: Addressing Acid Rain
Acid rain, primarily caused by the release of sulfur dioxide and nitrogen oxides into the atmosphere, poses a significant threat to ecosystems. Understanding the percent dissociation of acids contributing to acid rain is essential for assessing its environmental impact.
The acidity of rainwater influences the solubility and mobility of various pollutants in soil and water. Higher acidity, resulting from increased dissociation, can lead to the leaching of essential nutrients from soil and the release of toxic metals.
Predicting the effects of acid deposition requires modeling the dissociation behavior of weak acids in natural waters, considering factors such as temperature and ionic strength.
Pharmaceuticals: Optimizing Drug Delivery
In the pharmaceutical industry, the percent dissociation of a drug molecule significantly impacts its absorption, distribution, metabolism, and excretion (ADME) properties.
The ability of a drug to cross biological membranes, such as the intestinal lining, depends largely on its ionization state. Drugs that are largely un-ionized are generally more lipophilic and can diffuse more readily across cell membranes.
Understanding the pKa of a drug and the pH of different bodily compartments allows researchers to optimize drug formulations to maximize bioavailability – the fraction of the administered drug that reaches the systemic circulation. This knowledge is critical for ensuring efficacy and minimizing potential side effects.
Advanced Topics: Beyond the Basics
Having explored the fundamental principles and applications of percent dissociation, we now venture into more complex scenarios. These advanced topics build upon the foundational knowledge and offer a deeper understanding of acid-base equilibria in intricate chemical systems. We will explore the nuances of polyprotic acids, the influence of ionic strength, and spectroscopic methods used to determine percent dissociation.
Percent Dissociation in Polyprotic Acids
Polyprotic acids, such as sulfuric acid (H₂SO₄) and phosphoric acid (H₃PO₄), possess multiple ionizable protons. Unlike monoprotic acids, they undergo stepwise dissociation, each characterized by its own distinct dissociation constant (Ka1, Ka2, Ka3, etc.).
Understanding the percent dissociation of each proton in a polyprotic acid is crucial for accurately predicting its behavior in solution.
Each dissociation step is an equilibrium process, and the pH of the solution dictates the relative concentrations of the different protonated and deprotonated species.
Stepwise Dissociation and Equilibrium Constants
For a diprotic acid (H₂A), the dissociation occurs in two steps:
- H₂A ⇌ H⁺ + HA⁻ (Ka1)
- HA⁻ ⇌ H⁺ + A²⁻ (Ka2)
Generally, Ka1 > Ka2, indicating that the first proton is more readily dissociated than the second. This difference arises from the increased difficulty of removing a positive charge from a negatively charged species. The overall percent dissociation for each step must be considered independently, acknowledging that the second dissociation is influenced by the equilibrium established in the first.
Calculating Percent Dissociation for Each Step
Calculating the percent dissociation for each step requires careful consideration of the equilibrium constants and the initial concentration of the polyprotic acid. ICE tables can be used for each step.
However, the calculations become more complex because the concentration of the conjugate base from the first dissociation (HA⁻) affects the initial conditions for the second dissociation.
Approximations can sometimes simplify these calculations, particularly when the Ka values differ significantly (e.g., by a factor of 100 or more). In such cases, the second dissociation might be considered negligible compared to the first.
Ionic Strength Effects on Percent Dissociation
Ionic strength (I) is a measure of the total concentration of ions in a solution. It quantifies the effect of all ions on the equilibrium.
While introductory treatments often assume ideal behavior, real solutions, especially those with high ion concentrations, deviate from ideality. This deviation is due to the interactions between ions, which affect their activity, or effective concentration.
Activity Coefficients and the Debye-Hückel Theory
To account for non-ideal behavior, we introduce the concept of activity (a), which is related to concentration (c) by the activity coefficient (γ): a = γc. The activity coefficient reflects the deviation from ideal behavior.
The Debye-Hückel theory provides a framework for estimating activity coefficients based on ionic strength. According to this theory, increasing ionic strength generally decreases activity coefficients, particularly for ions with higher charges.
Impact on Acid-Base Equilibria
The effect of ionic strength on percent dissociation arises from its influence on the activity coefficients of the ions involved in the equilibrium.
For example, consider the dissociation of a weak acid (HA): HA ⇌ H⁺ + A⁻.
Increasing the ionic strength lowers the activity coefficients of H⁺ and A⁻, shifting the equilibrium to the right to compensate. This results in a higher degree of dissociation than predicted under ideal conditions.
Therefore, when working with solutions of high ionic strength (e.g., in biological systems or industrial processes), it is essential to consider activity coefficients to obtain accurate results.
Spectroscopic Methods for Determining Percent Dissociation
Spectroscopic techniques offer a powerful means of experimentally determining the percent dissociation of acids and bases. These methods rely on the fact that different protonated and deprotonated species often exhibit distinct spectral properties.
UV-Vis Spectroscopy
UV-Vis spectroscopy measures the absorption of ultraviolet and visible light by a substance.
If the acid (HA) and its conjugate base (A⁻) have different UV-Vis spectra, the ratio of their concentrations can be determined by measuring the absorbance at specific wavelengths.
By relating these concentrations to the initial concentration of the acid, the percent dissociation can be calculated.
NMR Spectroscopy
Nuclear Magnetic Resonance (NMR) spectroscopy provides detailed information about the structure and dynamics of molecules.
In acid-base systems, the chemical shifts of certain nuclei (e.g., ¹H, ¹³C) are sensitive to the protonation state of the molecule.
By analyzing the NMR spectrum, it is possible to determine the relative amounts of the protonated and deprotonated forms, thereby quantifying the percent dissociation.
Considerations for Spectroscopic Measurements
It's important to control experimental conditions such as temperature and pH, as these factors can influence the spectra.
Furthermore, calibration curves are often necessary to accurately relate absorbance or chemical shift to concentration. Spectroscopic methods provide valuable experimental data for validating theoretical calculations and gaining deeper insights into acid-base equilibria.
Having explored the intricate calculations and factors influencing percent dissociation, it's equally important to be aware of common pitfalls that can lead to inaccurate results. By identifying and understanding these mistakes, we can refine our approach and achieve a more precise understanding of acid-base equilibria.
Common Mistakes to Avoid
Calculating and interpreting percent dissociation involves several steps, each presenting opportunities for error. Recognizing these common mistakes is crucial for achieving accurate and reliable results. This section highlights the most frequent errors and provides guidance on how to avoid them, ensuring a solid grasp of acid-base chemistry principles.
Incorrect Use of the ICE Table
The ICE (Initial, Change, Equilibrium) table is an invaluable tool for calculating equilibrium concentrations and, consequently, percent dissociation. However, its misuse is a frequent source of error.
Common ICE Table Errors
-
Incorrect Initial Concentrations: Failing to accurately determine the initial concentrations of reactants and products is a primary error. Always double-check the problem statement and ensure correct units.
-
Improperly Defining 'Change' (x): The change in concentration ('x') must be defined correctly based on the stoichiometry of the balanced chemical equation. A mistake here propagates throughout the calculation.
-
Ignoring the Stoichiometry: Remember that changes in concentration are dictated by the stoichiometric coefficients in the balanced equation. For example, if the equation is A ⇌ 2B, the change in [B] is 2x, not x.
-
Algebraic Errors: Mistakes in solving for 'x' are common, especially when using the quadratic formula. Careful attention to detail is essential.
Best Practices for Using ICE Tables
- Write the balanced chemical equation clearly.
- Accurately list initial concentrations.
- Define the change ('x') based on stoichiometry.
- Express equilibrium concentrations in terms of 'x'.
- Double-check your algebraic solutions.
Ignoring Activity Coefficients at High Concentrations
At low concentrations, we often assume that activity coefficients are approximately equal to 1, simplifying calculations. However, this assumption breaks down at higher concentrations, where ionic interactions become significant.
The Impact of Ionic Strength
Ionic strength is a measure of the total concentration of ions in solution. High ionic strength leads to increased interionic attractions, which effectively reduce the "available" concentration of ions.
Activity Coefficients and Effective Concentration
Activity coefficients (γ) correct for these non-ideal behaviors. The effective concentration, or activity (a), is given by:
a = γ[Concentration]
Failing to account for activity coefficients at high concentrations can lead to significant errors in calculating percent dissociation.
When to Consider Activity Coefficients
- Concentrations above 0.1 M.
- Solutions containing multivalent ions.
Approximations and Limitations
While Debye-Hückel equation can be used to estimate activity coefficients, it's important to acknowledge that these are themselves approximations. For highly accurate results, experimental determination of activity coefficients may be necessary.
Misinterpreting pH and pKa Values
pH and pKa are fundamental concepts in acid-base chemistry, but their misuse can lead to incorrect conclusions about percent dissociation.
Understanding pH
pH is a measure of the acidity or basicity of a solution, defined as:
pH = -log[H⁺]
It's crucial to remember that pH reflects the hydrogen ion concentration at equilibrium, not necessarily the initial concentration of the acid.
Understanding pKa
pKa is a measure of the acid strength, defined as:
pKa = -log(Ka)
A lower pKa indicates a stronger acid, and vice versa.
The Henderson-Hasselbalch Equation
The Henderson-Hasselbalch equation relates pH, pKa, and the ratio of conjugate base to acid concentrations:
pH = pKa + log([A⁻]/[HA])
This equation is useful for estimating the ratio of conjugate base to acid at a given pH. However, it's essential to recognize its limitations:
- It's only accurate for buffer solutions.
- It assumes that activity coefficients are negligible.
Common Misinterpretations
- Assuming pH directly reflects percent dissociation: While related, pH is a measure of [H⁺], while percent dissociation reflects the extent of ionization.
- Equating pKa with pH: pKa is a constant for a given acid, while pH varies depending on concentration.
- Incorrectly applying the Henderson-Hasselbalch equation: Using the equation outside its valid range (i.e., not in a buffer solution).
Video: Mastering Percent Dissociation: A Complete Guide
FAQs: Mastering Percent Dissociation
Here are some frequently asked questions to help clarify your understanding of percent dissociation.
What exactly does "percent dissociation" tell me?
Percent dissociation tells you what percentage of a substance has broken down into its constituent ions or simpler molecules in a solution. It's a measure of the extent to which a substance ionizes or dissociates. A higher percent dissociation indicates a greater degree of ionization.
How does the initial concentration of a weak acid affect its percent dissociation?
Generally, the percent dissociation of a weak acid increases as its initial concentration decreases. This is because the equilibrium shifts towards dissociation as the concentration becomes more dilute, maintaining the equilibrium constant (Ka).
What's the difference between degree of dissociation and percent dissociation?
The degree of dissociation is the fraction of the substance that has dissociated, while percent dissociation is simply the degree of dissociation multiplied by 100 to express it as a percentage. They represent the same information, just in different formats.
Can percent dissociation be greater than 100%?
No, percent dissociation cannot be greater than 100%. A percent dissociation of 100% indicates that all of the substance has dissociated into its ions or components. It's a representation of the portion of the initial substance that has undergone dissociation.
