Change in Kinetic Energy Formula: A Practical Guide

The exploration of energy dynamics within physics often leads to the application of the change in kinetic energy formula, where the initial velocity and final velocity significantly impact the resulting energy differential. Understanding this formula is crucial for engineers at institutions like MIT, who frequently utilize it in designing mechanical systems to predict performance under various operational conditions. Furthermore, tools such as MATLAB can assist in these calculations, providing simulations that refine theoretical predictions against empirical data, ensuring alignment with real-world scenarios. These practical applications underscore the formula's importance, as emphasized by physicists like James Prescott Joule in his foundational work on energy conservation and transformation.

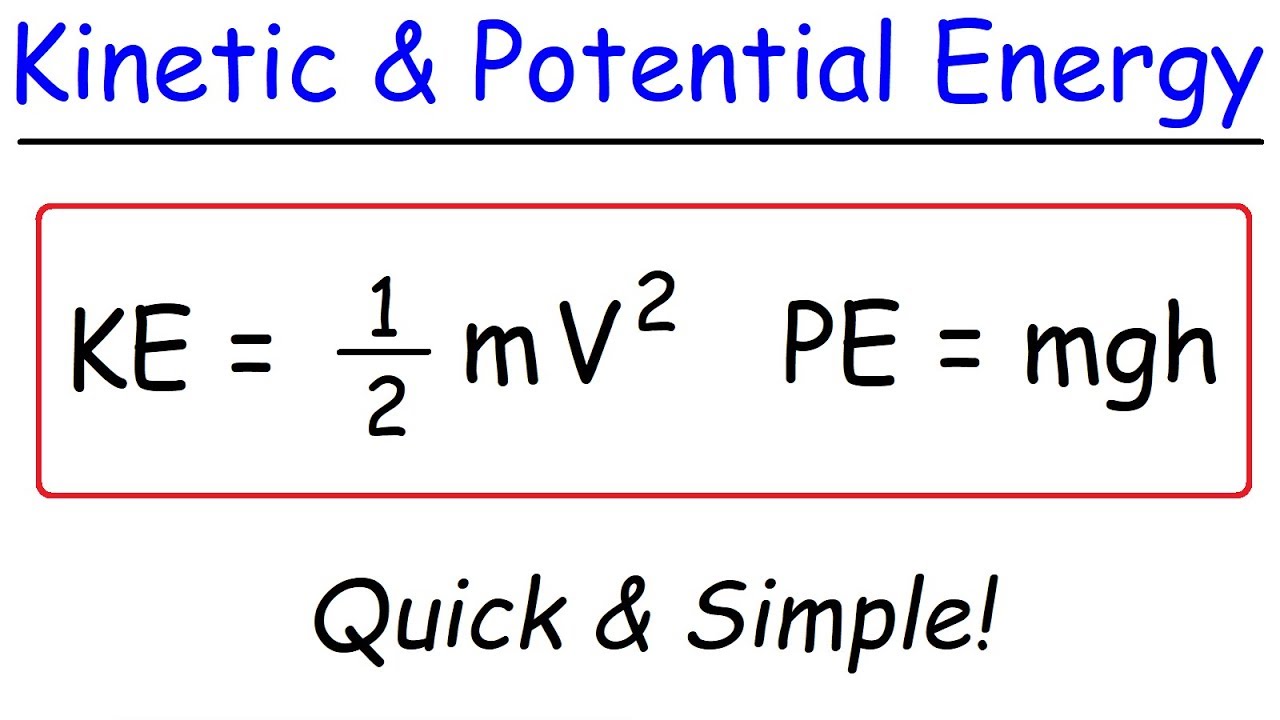
Image taken from the YouTube channel The Organic Chemistry Tutor , from the video titled Kinetic Energy and Potential Energy .

Kinetic energy, often abbreviated as KE, is a cornerstone concept in physics.

It quantifies the energy possessed by an object due to its motion.

Understanding kinetic energy is fundamental to grasping how the world around us works.

The Significance of Kinetic Energy

The principles of kinetic energy are not confined to textbooks or laboratories.

They are omnipresent, governing everything from the simple act of walking to the complex dynamics of celestial bodies.

In classical mechanics, KE helps to explain the motion of objects under the influence of forces.

It allows us to predict how objects will behave.

Beyond physics, kinetic energy plays a crucial role in engineering, sports, transportation, and even weather forecasting.

Key Components: Mass, Velocity, and the ΔKE Formula

Several key components are essential for understanding kinetic energy.

Mass is a measure of an object's inertia, its resistance to changes in motion.

Velocity describes both the speed and direction of an object's movement.

Finally, the change in kinetic energy (ΔKE) formula allows us to calculate how much an object's kinetic energy changes as its velocity changes.

These components are interconnected and vital for analyzing motion in a variety of contexts.

Key Concepts: Mass, Velocity, and the Scalar Nature of Kinetic Energy

With the groundwork laid, we now turn our attention to the fundamental building blocks that govern kinetic energy.

Understanding these concepts – mass, velocity, and the scalar nature of KE – is critical for mastering the ΔKE formula and applying it effectively.

Moreover, familiarity with their respective units is essential for accurate calculations and meaningful interpretations.

Mass: A Measure of Inertia

In physics, mass (m) is not merely a measure of how much "stuff" is in an object.

Instead, mass is a quantification of an object's inertia.

Inertia, in turn, is the resistance an object exhibits to changes in its state of motion.

A more massive object has greater inertia, meaning it requires a larger force to accelerate it or to bring it to a stop.

Consider a bowling ball versus a tennis ball.

The bowling ball, with its greater mass, is significantly harder to accelerate or decelerate than the tennis ball.

This difference in resistance to motion directly reflects the difference in their inertial properties.

Velocity: Rate of Change of Position

While mass characterizes an object's resistance to motion, velocity (v) describes the motion itself.

More precisely, velocity is the rate at which an object's position changes with respect to time.

It's a vector quantity, possessing both magnitude (speed) and direction.

Two cars moving at the same speed but in opposite directions have different velocities.

When analyzing kinetic energy, it's important to consider only the magnitude of the velocity (i.e., the speed) because direction is irrelevant.

However, understanding the vector nature of velocity is still essential for a complete description of motion.

Kinetic Energy: A Scalar Quantity

Kinetic energy, unlike velocity, is a scalar quantity.

This means that it has magnitude but no direction.

The kinetic energy of an object depends only on its mass and speed, regardless of the direction of its motion.

Imagine two identical objects moving at the same speed, one heading east and the other west.

Even though their velocities are different (due to opposite directions), their kinetic energies are the same.

This scalar nature simplifies many calculations, as we only need to consider the speed of the object, not its direction of travel.

Units of Measurement

Consistent units are paramount in physics calculations, and kinetic energy is no exception.

In the International System of Units (SI), mass is measured in kilograms (kg), and speed is measured in meters per second (m/s).

When these units are used in the kinetic energy formula (KE = 1/2 m v^2), the resulting kinetic energy is expressed in Joules (J).

One Joule is defined as the amount of work done when a force of one Newton displaces an object one meter in the direction of the force (1 J = 1 N⋅m = 1 kg⋅m²/s²).

Maintaining consistency in units ensures that calculations are accurate and that the results are physically meaningful.

Unveiling the Change in Kinetic Energy (ΔKE) Formula

The concept of kinetic energy provides a snapshot of an object's energy state at a specific instant. However, in many scenarios, we're more interested in how kinetic energy changes over time. This is where the Change in Kinetic Energy (ΔKE) becomes crucial. It is a tool that allows us to understand the dynamic energy transformations occurring during motion.

Change in Kinetic Energy (ΔKE): A Definition

The Change in Kinetic Energy (ΔKE) represents the difference between an object's final kinetic energy (KEf) and its initial kinetic energy (KEi). This difference, ΔKE = KEf - KEi, tells us how much the object's kinetic energy has increased or decreased.

A positive ΔKE signifies that the object has gained kinetic energy, meaning its speed has increased. Conversely, a negative ΔKE indicates a loss of kinetic energy, corresponding to a decrease in speed.

Presenting the ΔKE Formula

The formula to calculate the change in kinetic energy is:

ΔKE = 1/2 m (vf^2 - vi^2)

Where:

• ΔKE represents the change in kinetic energy, measured in Joules (J).
• m represents the mass of the object, measured in kilograms (kg).
• vf represents the final velocity of the object, measured in meters per second (m/s).
• vi represents the initial velocity of the object, measured in meters per second (m/s).

This formula effectively calculates the final kinetic energy (1/2 m vf^2) and subtracts the initial kinetic energy (1/2 m vi^2).

Formula Breakdown: Understanding the Components

Let's dissect the ΔKE formula to gain a deeper understanding of its components and how they interact.

Final Velocity (vf) and Initial Velocity (vi)

The final velocity (vf) is the object's velocity at the end of the time interval under consideration. The initial velocity (vi) is the object's velocity at the beginning of that same time interval. Both are crucial for determining the change in kinetic energy.

It's the difference between the squares of these velocities (vf^2 - vi^2) that matters most. This emphasizes that the change in kinetic energy is more sensitive to changes in speed at higher velocities.

A Step-by-Step Approach to Using the Formula

To effectively use the ΔKE formula, follow these steps:

1. Identify the knowns: Determine the mass (m) of the object, its initial velocity (vi), and its final velocity (vf). Ensure all values are in SI units (kg, m/s).
2. Square the velocities: Calculate vf^2 (final velocity squared) and vi^2 (initial velocity squared).
3. Find the difference: Subtract the initial velocity squared from the final velocity squared: (vf^2 - vi^2).
4. Multiply by mass and 1/2: Multiply the result from step 3 by the mass (m) of the object and then by 1/2 (or 0.5).
5. State the result: The final result is the change in kinetic energy (ΔKE), expressed in Joules (J).

By meticulously following these steps, you can accurately calculate the change in kinetic energy for a wide variety of scenarios. Understanding the formula's components and applying it systematically are the keys to success.

The Work-Energy Theorem: Connecting Work and Kinetic Energy

Having established the Change in Kinetic Energy (ΔKE) formula, it's imperative to understand how this change is brought about. This is where the Work-Energy Theorem becomes invaluable. It provides a direct link between the work done on an object and the resultant change in its kinetic energy. In essence, it clarifies work as the primary agent responsible for altering an object's state of motion.

Defining Work in the Context of Energy Transfer

Work (W), in physics, is defined as the energy transferred to or from an object by a force acting upon it. This transfer occurs when a force causes a displacement of the object. The amount of work done depends on the magnitude of the force, the distance over which it acts, and the angle between the force and the displacement.

Mathematically, work is expressed as:

W = F d cos(θ)

Where:

• W is the work done (measured in Joules, J).
• F is the magnitude of the force (measured in Newtons, N).
• d is the magnitude of the displacement (measured in meters, m).
• θ is the angle between the force and displacement vectors.

The Work-Energy Theorem: A Formal Statement

The Work-Energy Theorem succinctly states: The net work done on an object is equal to the change in its kinetic energy. This can be expressed by the deceptively simple, yet profoundly important equation:

W = ΔKE

This equation reveals that work isn't just some abstract concept, but rather the direct cause of changes in an object's kinetic energy. A positive work value indicates an increase in kinetic energy (acceleration), while a negative work value indicates a decrease in kinetic energy (deceleration).

Work as the Cause of Kinetic Energy Change: Conceptual Examples

To solidify this understanding, let's consider a few conceptual examples:

• Pushing a Box: Imagine pushing a box across a floor. The force you apply over the distance the box moves constitutes work. This work directly translates into an increase in the box's kinetic energy, causing it to accelerate from rest.
• Braking a Car: When you apply the brakes in a car, the friction between the brake pads and the rotors exerts a force that opposes the car's motion. This force does negative work on the car, causing its kinetic energy to decrease and the car to slow down. The heat generated in the brakes is a manifestation of this energy dissipation.
• Lifting an Object: When lifting an object vertically, you apply an upward force to counteract gravity. The work you do increases the object's potential energy (covered later), but if the object is also gaining speed as you lift it, some of the work is also increasing its kinetic energy.

These examples demonstrate that work is the fundamental mechanism responsible for altering an object's kinetic energy. It is the bridge connecting force, displacement, and the dynamic world of motion.

Practical Applications: Calculating ΔKE in Real-World Scenarios

The Change in Kinetic Energy (ΔKE) formula isn't just a theoretical construct; it's a powerful tool for analyzing motion in the real world. Let's explore how we can apply the formula to understand and quantify changes in kinetic energy in everyday situations.

ΔKE and Moving Vehicles: A Worked Example

Consider a car with a mass of 1500 kg accelerating from rest to a speed of 25 m/s (approximately 56 mph). We can use the ΔKE formula to determine the change in kinetic energy of the car during this acceleration.

Step-by-Step Calculation:

1. Identify the known variables:

   • Mass (m) = 1500 kg
   • Initial velocity (vi) = 0 m/s (since the car starts from rest)
   • Final velocity (vf) = 25 m/s

2. Apply the ΔKE formula:

   • ΔKE = 1/2 m (vf^2 - vi^2)
   • ΔKE = 1/2 1500 kg ((25 m/s)^2 - (0 m/s)^2)
   • ΔKE = 1/2 1500 kg (625 m^2/s^2)
   • ΔKE = 468750 J

Therefore, the change in kinetic energy of the car is 468,750 Joules. This is the amount of energy that the car's engine had to supply to bring it up to that speed. This highlights how directly ΔKE quantifies the energy involved in changes of motion.

Analyzing Kinetic Energy Change in a Falling Object

Now, let's turn our attention to a falling object. Consider a 1 kg ball dropped from a height of 10 meters. Neglecting air resistance, we can determine the ball's change in kinetic energy just before it hits the ground.

Determining Final Velocity:

First, we need to calculate the final velocity (vf) of the ball just before impact. We can use the following kinematic equation, derived from the principles of uniformly accelerated motion:

vf^2 = vi^2 + 2 g Δy

Where:

• vi = Initial velocity (0 m/s, since the ball is dropped)
• g = Acceleration due to gravity (approximately 9.8 m/s^2)
• Δy = Change in vertical position (10 m)

Plugging in the values:

• vf^2 = 0^2 + 2 9.8 m/s^2 10 m
• vf^2 = 196 m^2/s^2
• vf = √(196 m^2/s^2) = 14 m/s

Calculating ΔKE:

Now that we know the final velocity, we can calculate the change in kinetic energy:

• ΔKE = 1/2 m (vf^2 - vi^2)
• ΔKE = 1/2 1 kg ((14 m/s)^2 - (0 m/s)^2)
• ΔKE = 1/2 1 kg (196 m^2/s^2)
• ΔKE = 98 J

Therefore, the change in kinetic energy of the ball just before impact is 98 Joules. This amount precisely matches the potential energy the ball lost during its fall, illustrating the conversion between potential and kinetic energy.

Additional Applications of the ΔKE Formula

The applications of the ΔKE formula extend far beyond these examples. It can be used to analyze the kinetic energy changes in various scenarios, including:

• Roller coasters: Calculating the kinetic energy of a roller coaster car at different points along the track.
• Sports: Analyzing the kinetic energy of a baseball as it's pitched or the kinetic energy of a runner as they sprint.
• Rotating Machinery: Assessing the change in kinetic energy of a spinning flywheel or turbine.

By understanding and applying the ΔKE formula, we can gain valuable insights into the dynamic world around us. It is a fundamental tool for understanding and quantifying motion, making it an essential concept in physics and engineering.

Avoiding Common Mistakes and Understanding Key Assumptions

Calculating the Change in Kinetic Energy (ΔKE) can seem straightforward, but subtle errors and overlooked assumptions can lead to inaccurate results. Mastering the formula requires not only understanding how to apply it, but also what not to do and when the formula is most applicable. This section highlights common pitfalls and outlines essential assumptions to ensure accurate and meaningful calculations.

Common Mistakes to Avoid

Several frequent errors can creep into ΔKE calculations, compromising the validity of the results. Being aware of these mistakes is the first step towards avoiding them.

Forgetting to Square Velocities

The ΔKE formula, ΔKE = 1/2 m (vf^2 - vi^2), explicitly involves squaring the final (vf) and initial (vi) velocities. This squaring operation is crucial; failing to do so will drastically underestimate the kinetic energy change.

Remember that kinetic energy is proportional to the square of the velocity. Therefore, an error in squaring the velocities will have a disproportionately large impact on the final result.

Using Inconsistent or Incorrect Units

Maintaining consistent units throughout the calculation is paramount. The standard units are kilograms (kg) for mass, meters per second (m/s) for velocity, and Joules (J) for energy.

Mixing units (e.g., using grams for mass while velocities are in m/s) will produce nonsensical results. Always convert all quantities to the standard units before applying the ΔKE formula. Dimensional analysis can be helpful in verifying that all units are consistent.

Using incorrect units is equally problematic, for example, miles per hour (mph) for velocity without converting it to meters per second (m/s).

Key Assumptions and Their Implications

The ΔKE formula, like any physics equation, relies on certain assumptions. Recognizing these assumptions and understanding their limitations is critical for interpreting the results accurately.

Neglecting Air Resistance or Friction

In many introductory physics problems, air resistance and friction are conveniently ignored to simplify the calculations. However, in real-world scenarios, these forces are often significant.

Air resistance and friction dissipate energy from the system, meaning that not all the work done on an object will translate directly into a change in kinetic energy. This means the calculated ΔKE will not reflect the actual energy input.

In situations where air resistance or friction is significant, more advanced techniques (often involving energy conservation with non-conservative forces) are required to obtain accurate results.

Assuming a Constant Mass During Motion

The standard ΔKE formula assumes that the mass of the object remains constant throughout its motion. This assumption is valid in most everyday scenarios, such as a car accelerating or a ball being thrown.

However, there are situations where the mass changes significantly during motion, such as a rocket expelling fuel or a conveyor belt dropping off material. In these cases, the standard ΔKE formula is not directly applicable.

Calculations involving variable mass require a more sophisticated approach, often involving calculus and the principles of momentum conservation.

Kinetic Energy, Potential Energy, and the Conservation of Energy

The narrative of motion wouldn't be complete without considering the interplay between kinetic energy (KE) and potential energy (PE). These two forms of energy are inextricably linked, especially when viewed through the lens of the conservation of energy principle. Understanding this relationship provides a more profound appreciation of how energy behaves and transforms within physical systems.

Understanding Potential Energy

Potential energy (PE) represents the energy an object possesses due to its position or condition. Unlike kinetic energy, which is associated with motion, potential energy is stored energy with the potential to be converted into other forms, including kinetic energy. Several types of potential energy exist, each dependent on the specific forces at play.

Gravitational potential energy, perhaps the most commonly encountered form, depends on an object's height above a reference point. A raised object has the potential to do work as it falls due to gravity.

Elastic potential energy is stored in deformable objects, like springs or rubber bands, when they are stretched or compressed. The amount of stored energy depends on the object's stiffness and the amount of deformation.

The Principle of Conservation of Energy

At the heart of physics lies the fundamental principle of conservation of energy. This principle states that the total energy within an isolated system remains constant over time. Energy cannot be created or destroyed; it can only be transformed from one form to another. This is a cornerstone of physics and has profound implications for understanding all physical phenomena.

In practical terms, this means that in a closed system (one where no energy enters or leaves), the sum of all forms of energy – kinetic, potential, thermal, etc. – will always remain the same. Changes in one form of energy must be accompanied by corresponding changes in other forms to maintain the overall energy balance.

Kinetic and Potential Energy Transformations

The dynamic relationship between kinetic and potential energy is best illustrated through examples where energy continuously transforms between these two forms. Consider a pendulum swinging back and forth.

At the highest point of its swing, the pendulum momentarily comes to rest. At this point, its kinetic energy is zero, and its gravitational potential energy is at a maximum. As the pendulum swings downward, its potential energy is converted into kinetic energy, causing it to accelerate.

At the bottom of its swing, the pendulum reaches its maximum speed, and its kinetic energy is at its highest. Its potential energy is at its minimum (assuming the bottom point is the reference level). As the pendulum swings upward on the other side, the kinetic energy is converted back into potential energy, slowing the pendulum down until it reaches its highest point again, completing the cycle. The total mechanical energy (KE + PE) remains nearly constant throughout the swing (ignoring small losses due to air resistance and friction).

Another classic example is a falling object. Initially, at a certain height, the object possesses gravitational potential energy. As it falls, this potential energy is converted into kinetic energy, causing its velocity to increase. Just before impact, almost all of the initial potential energy has been transformed into kinetic energy.

Understanding these transformations and the conservation of energy allows physicists and engineers to predict and analyze the behavior of complex systems, from simple mechanical devices to intricate cosmological phenomena.

Factors Influencing Kinetic Energy Change

Changes in kinetic energy don't occur in a vacuum. External factors are the driving forces behind these changes, directly influencing an object's motion and, consequently, its kinetic energy. Understanding these factors is crucial for a comprehensive understanding of energy dynamics. Among the most important are acceleration and net force.

The Role of Acceleration

Acceleration is the rate of change of velocity. It's the direct link between forces and motion. When an object accelerates, its velocity changes, and, as a direct consequence, its kinetic energy changes. The relationship is quite straightforward: acceleration increases kinetic energy when in the direction of motion, and decreases it when it opposes the motion.

Acceleration and Increasing Kinetic Energy

If an object experiences acceleration in the same direction as its velocity, its speed increases. This increase in speed directly translates to an increase in kinetic energy. Imagine a car accelerating from a standstill. The engine provides a force, resulting in acceleration, and the car's kinetic energy continuously increases as it gains speed.

Acceleration and Decreasing Kinetic Energy

Conversely, if an object experiences acceleration in the opposite direction to its velocity (deceleration), its speed decreases. This decrease in speed directly leads to a decrease in kinetic energy. Consider a car braking: the brakes apply a force opposite to the direction of motion, causing deceleration and a reduction in kinetic energy.

The Impact of Net Force

Net force is the vector sum of all forces acting on an object. It dictates the overall force that influences the object's motion. A greater net force results in a greater acceleration, which, as we've established, directly impacts the change in kinetic energy.

Force and Kinetic Energy: A Direct Correlation

The relationship between net force and kinetic energy is closely tied to Newton's Second Law of Motion (F = ma). This law dictates that the net force acting on an object is equal to its mass times its acceleration. Consequently, a larger net force will generate a larger acceleration, and the greater the acceleration, the larger the change in kinetic energy, assuming the mass remains constant.

Examples of Net Force Influencing Kinetic Energy

Think about pushing a box across a floor. The harder you push (the greater the net force), the faster the box accelerates, and the more rapidly its kinetic energy increases. Conversely, if friction is a significant force opposing the motion, the net force is reduced, the acceleration is less, and the kinetic energy increases at a slower rate.

In essence, both acceleration and net force are intertwined in governing changes in kinetic energy. Acceleration is the direct manifestation of the change in velocity, while net force is the cause of that acceleration.

Video: Change in Kinetic Energy Formula: A Practical Guide

Play Video

So, next time you're trying to figure out how much energy something's gained or lost in motion, remember the change in kinetic energy formula (ΔKE = 1/2 m (vf^2 - vi^2)). It might seem intimidating at first, but break it down, and you'll be calculating kinetic energy changes like a pro in no time. Happy calculating!