Percent Dissociation Formula: A Complete Guide

The percent dissociation formula serves as a cornerstone in quantitative chemical analysis, specifically within the realm of acid-base chemistry. Its primary application lies in determining the extent to which a weak acid or base dissociates into its constituent ions in a solution. For instance, the concentration of acetic acid, a weak acid, undergoing dissociation can be precisely quantified using this formula. Arrhenius acids and bases, characterized by their behavior in aqueous solutions, are commonly analyzed using the percent dissociation formula to understand their ionization properties. Laboratories equipped with titration setups and pH meters routinely employ the percent dissociation formula to derive meaningful insights from experimental data.

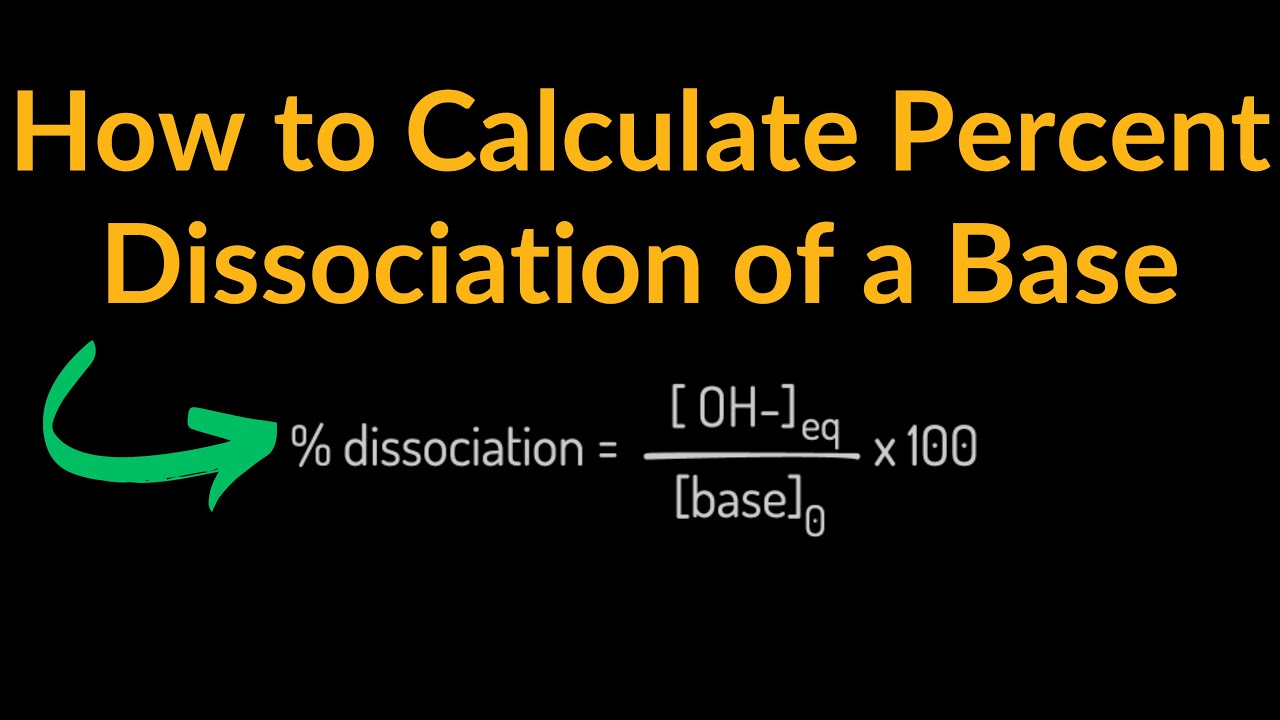
Image taken from the YouTube channel Conquer Chemistry , from the video titled How to Calculate the Percent Dissociation of a Base Shortcut, Examples, Practice Problems, Equation .

Chemical dissociation, a fundamental process in chemistry, dictates the behavior of substances dissolved in water. It is the process where a molecule separates into ions or radicals when dissolved in a solution. Understanding this phenomenon is not merely an academic exercise.

It's a cornerstone for comprehending a vast array of chemical, biological, and environmental processes. From the intricate mechanisms of enzyme catalysis to the large-scale dynamics of ocean acidification, dissociation plays a critical role.

Defining Dissociation: The Breakdown of Molecules

Dissociation, at its core, involves the breaking of chemical bonds within a molecule. This leads to the formation of two or more new chemical species. These species are typically ions carrying a positive or negative charge, or radicals containing an unpaired electron.

When the solvent is water, we specifically refer to this process as dissociation in aqueous solution. The solvent's polar nature significantly impacts the dissociation process. It stabilizes the resulting ions or radicals through solvation.

The Significance of Dissociation Across Disciplines

The implications of understanding dissociation extend far beyond the chemistry laboratory. In biology, for instance, the dissociation of acids and bases within cellular fluids is essential for maintaining pH balance and enabling enzymatic activity.

In environmental science, the dissociation of pollutants in aquatic ecosystems determines their mobility, toxicity, and ultimate fate. Without a firm grasp on dissociation principles, we would be ill-equipped to address critical environmental challenges.

Chemical engineers rely on dissociation data to optimize reaction conditions. Pharmaceutical scientists use dissociation constants to design effective drug delivery systems.

Equilibrium and Acid-Base Chemistry: Foundational Pillars

Two key concepts underpin the understanding of chemical dissociation: chemical equilibrium and acid-base chemistry. Chemical equilibrium describes the dynamic state where the rates of the forward and reverse reactions are equal. This results in no net change in the concentrations of reactants and products.

Dissociation reactions are almost always equilibrium processes. The extent to which a substance dissociates is governed by the equilibrium constant. It dictates the relative amounts of undissociated molecules and dissociated ions at equilibrium.

Acid-base chemistry introduces the concepts of proton donors (acids) and proton acceptors (bases). Many dissociation reactions involve the transfer of protons between molecules and water.

Core Concepts: Deconstructing Dissociation

Chemical dissociation, a fundamental process in chemistry, dictates the behavior of substances dissolved in water. It is the process where a molecule separates into ions or radicals when dissolved in a solution. Understanding this phenomenon is not merely an academic exercise. It's a cornerstone for comprehending a vast array of chemical, biological, and environmental processes. To truly grasp the intricacies of dissociation, it's essential to deconstruct the underlying concepts that govern this behavior.

Strong vs. Weak Electrolytes: A Tale of Two Dissociations

Electrolytes, substances that conduct electricity when dissolved in water, owe their conductivity to the presence of ions. However, not all electrolytes are created equal.

Strong electrolytes dissociate completely into ions when dissolved. Classic examples include strong acids like hydrochloric acid (HCl) and strong bases like sodium hydroxide (NaOH), as well as many soluble salts. Their solutions are characterized by a high concentration of ions, leading to excellent electrical conductivity.

In stark contrast, weak electrolytes only partially dissociate in solution. This means that at equilibrium, a significant portion of the substance remains in its undissociated form. Weak acids, such as acetic acid (CH3COOH), and weak bases, such as ammonia (NH3), exemplify this behavior. The limited ion concentration in their solutions results in lower electrical conductivity compared to strong electrolytes.

Degree of Dissociation (α): Quantifying the Extent

The degree of dissociation (α) provides a quantitative measure of the extent to which a substance dissociates in solution. It is defined as the ratio of the number of moles of the substance that have dissociated to the total number of moles of the substance initially dissolved.

α = (Moles dissociated) / (Total moles initially dissolved)

The value of α ranges from 0 to 1, where 0 indicates no dissociation and 1 indicates complete dissociation. The degree of dissociation is influenced by several factors, including:

• Nature of the electrolyte: Strong electrolytes have α ≈ 1, while weak electrolytes have α << 1.
• Concentration: For weak electrolytes, the degree of dissociation generally increases with decreasing concentration.
• Temperature: The effect of temperature on α depends on whether the dissociation process is endothermic or exothermic.

Equilibrium Constant (K): Predicting Reaction Extent

Chemical reactions, including dissociation, often reach a state of equilibrium where the rates of the forward and reverse reactions are equal. The equilibrium constant (K) is a numerical value that expresses the ratio of products to reactants at equilibrium.

For a general dissociation reaction:

AB ⇌ A+ + B-

The equilibrium constant is expressed as:

K = [A+][B-] / [AB]

A large value of K indicates that the equilibrium lies to the right, favoring the formation of products (i.e., a high degree of dissociation). Conversely, a small value of K indicates that the equilibrium lies to the left, favoring the reactants (i.e., a low degree of dissociation).

Dissociation Constants (Ka, Kb): Acids, Bases, and Their Strengths

For acids and bases, specific dissociation constants are used to quantify their strength. Ka represents the acid dissociation constant, while Kb represents the base dissociation constant. These constants reflect the extent to which an acid donates a proton (H+) or a base accepts a proton when dissolved in water.

For a weak acid HA:

HA + H2O ⇌ H3O+ + A-

Ka = [H3O+][A-] / [HA]

For a weak base B:

B + H2O ⇌ BH+ + OH-

Kb = [BH+][OH-] / [B]

Higher Ka values indicate a stronger acid, while higher Kb values indicate a stronger base. These constants are crucial for predicting the pH of solutions and understanding acid-base reactions.

Percent Dissociation: A Practical Application

Percent dissociation is another way to express the extent of dissociation. It is calculated by multiplying the degree of dissociation (α) by 100%.

Percent Dissociation = α * 100%

This value provides a readily interpretable measure of the percentage of the substance that has dissociated in solution. For example, if a weak acid has a percent dissociation of 5%, it means that only 5% of the acid molecules have dissociated into ions.

Example:

Calculate the percent dissociation of a 0.10 M solution of acetic acid (Ka = 1.8 x 10-5).

1. Set up an ICE table.
2. Calculate α using the Ka expression.
3. Multiply α by 100% to obtain the percent dissociation.

Concentration: The Quantitative Foundation

Concentration is a fundamental concept in quantitative analysis, referring to the amount of a substance (solute) present in a given volume of solution. Molarity (M), defined as moles of solute per liter of solution, is a commonly used unit of concentration.

Molarity (M) = (Moles of solute) / (Liters of solution)

Understanding concentration is essential for calculating equilibrium constants, predicting reaction rates, and performing stoichiometric calculations.

Electrolytes: The Conductors of Dissociation

Electrolytes are substances that produce ions when dissolved in a solvent, such as water, and consequently form an electrically conducting medium. The process by which electrolytes form ions is called ionization.

Electrolytes can be strong, weak, or non-electrolytes depending on their ability to dissociate into ions. Strong electrolytes dissociate completely, weak electrolytes dissociate partially, and non-electrolytes do not dissociate at all. The presence and concentration of ions in an electrolyte solution directly influence its electrical conductivity.

Stoichiometry: The Foundation for Calculations

Stoichiometry plays a crucial role in understanding and quantifying dissociation processes. It provides the numerical relationships between reactants and products in a chemical reaction.

By applying stoichiometric principles, we can determine the concentrations of ions produced during dissociation, calculate equilibrium constants, and predict the outcome of chemical reactions. Understanding the stoichiometric ratios in a dissociation reaction is essential for accurate calculations and meaningful interpretations.

Tools and Techniques: Quantifying Dissociation in the Lab

Chemical dissociation, a fundamental process in chemistry, dictates the behavior of substances dissolved in water. It is the process where a molecule separates into ions or radicals when dissolved in a solution. Understanding this phenomenon is not merely an academic exercise. It's a cornerstone for comprehensive analysis. Thus, quantifying dissociation becomes paramount. We will discuss the practical tools and techniques that enable us to analyze and quantify this process effectively in the laboratory.

The ICE Table Method: A Step-by-Step Guide

The ICE (Initial, Change, Equilibrium) table is an indispensable tool for solving equilibrium problems related to dissociation. It provides a structured approach to tracking concentrations as a reaction progresses towards equilibrium.

1. Setting Up the Table: Create a table with rows labeled "Initial," "Change," and "Equilibrium." Columns should correspond to each reactant and product in the balanced chemical equation.

2. Initial Concentrations: Fill in the "Initial" row with the initial concentrations of all species. If a species is not initially present, its concentration is zero.

3. Change in Concentrations: Use the variable 'x' to represent the change in concentration as the reaction proceeds. The sign of 'x' is determined by the stoichiometry of the balanced equation; reactants decrease (-x), and products increase (+x).

4. Equilibrium Concentrations: Calculate the "Equilibrium" row by adding the "Initial" and "Change" rows. These values represent the concentrations of each species at equilibrium in terms of 'x'.

5. Solving for 'x': Substitute the equilibrium concentrations into the equilibrium constant expression (Ka, Kb) and solve for 'x'. This often involves solving a quadratic equation or, under certain conditions, using approximations.

6. Calculating Equilibrium Concentrations: Once 'x' is known, plug its value back into the "Equilibrium" row to find the equilibrium concentrations of all species.

Example: Consider the dissociation of a weak acid, HA, in water: HA(aq) ⇌ H+(aq) + A-(aq). Suppose the initial concentration of HA is 0.1 M and Ka = 1.8 x 10-5. Constructing an ICE table allows you to solve for [H+], [A-], and [HA] at equilibrium.

pH Measurement: Gauging Acidity and Basicity

pH, a measure of the acidity or basicity of a solution, is defined as the negative logarithm (base 10) of the hydronium ion concentration: pH = -log[H3O+]. The pH scale typically ranges from 0 to 14, with values below 7 indicating acidic solutions, values above 7 indicating basic solutions, and a value of 7 indicating a neutral solution.

pH and Ion Concentrations

pH is inversely related to both hydronium (H3O+) and hydroxide (OH-) ion concentrations.

• In acidic solutions, [H3O+] > [OH-], resulting in a pH < 7.
• In basic solutions, [H3O+] < [OH-], resulting in a pH > 7.
• In neutral solutions, [H3O+] = [OH-], resulting in a pH = 7.

pH Meters: Precision Instruments

pH meters are electronic instruments used to measure pH accurately. They consist of a pH-sensitive electrode and a reference electrode. These meters provide a digital readout of the pH, making them essential tools in any chemistry laboratory.

Le Chatelier's Principle: Predicting Equilibrium Shifts

Le Chatelier's Principle states that if a change of condition is applied to a system in equilibrium, the system will shift in a direction that relieves the stress. These conditions include changes in temperature, pressure, or concentration.

• Temperature: For an endothermic reaction, increasing the temperature shifts the equilibrium towards the products. For an exothermic reaction, increasing the temperature shifts the equilibrium towards the reactants.

  You also like

  Was Ist Das? German Phrases for American Travel

• Pressure: Changes in pressure primarily affect gaseous systems. Increasing the pressure shifts the equilibrium towards the side with fewer moles of gas.

• Concentration: Increasing the concentration of a reactant shifts the equilibrium towards the products, and vice versa.

Example: Consider the dissociation of a weak base, B, in water: B(aq) + H2O(l) ⇌ BH+(aq) + OH-(aq). Adding OH- ions to the solution will shift the equilibrium to the left, decreasing the dissociation of B and reducing the concentration of BH+.

Leveraging Calculators for Efficient Problem Solving

Calculators are indispensable for solving complex dissociation problems. They facilitate the quick and accurate computation of equilibrium concentrations, pH values, and dissociation constants. Programmable calculators can be particularly useful for repetitive calculations or solving complex equations.

Advanced Applications: Exploring the Nuances of Dissociation

Chemical dissociation, a fundamental process in chemistry, dictates the behavior of substances dissolved in water. It is the process where a molecule separates into ions or radicals when dissolved in a solution. Understanding this phenomenon is not merely an academic exercise. It's a cornerstone in various scientific disciplines. Now, building upon the foundational principles and analytical techniques, we delve into the complexities and advanced applications of dissociation.

Temperature's Influence on Dissociation Equilibrium

Temperature plays a critical role in influencing the equilibrium of dissociation reactions. Generally, for endothermic dissociation processes, an increase in temperature favors the dissociation of the solute, shifting the equilibrium towards the products.

Conversely, exothermic dissociation processes are favored by lower temperatures.

The quantitative relationship between temperature and the equilibrium constant, K, is described by the Van't Hoff equation:

ln(K₂/K₁) = -ΔH°/R (1/T₂ - 1/T₁)

Where:

• K₁ and K₂ are the equilibrium constants at temperatures T₁ and T₂, respectively.
• ΔH° is the standard enthalpy change of the reaction.
• R is the ideal gas constant.

This equation highlights that the temperature dependence of the equilibrium constant is directly related to the enthalpy change of the reaction. It provides a powerful tool for predicting how changes in temperature will affect the extent of dissociation.

The Common Ion Effect

The common ion effect describes the decrease in the solubility of a sparingly soluble salt when a soluble salt containing a common ion is added to the solution. This effect is a direct consequence of Le Chatelier's principle.

Consider the dissolution of silver chloride (AgCl), a sparingly soluble salt:

AgCl(s) ⇌ Ag⁺(aq) + Cl⁻(aq)

If we add a soluble chloride salt, such as NaCl, to this solution, the concentration of Cl⁻ ions increases. According to Le Chatelier's principle, the equilibrium will shift to the left, reducing the solubility of AgCl.

The common ion effect is crucial in controlling the solubility of ionic compounds and is widely applied in analytical chemistry and pharmaceutical formulations.

Dissociation in Polyprotic Acids

Polyprotic acids, such as sulfuric acid (H₂SO₄) and phosphoric acid (H₃PO₄), can donate more than one proton (H⁺) per molecule. The dissociation of these acids occurs in a stepwise manner, with each step characterized by its own acid dissociation constant (Kₐ).

For example, phosphoric acid (H₃PO₄) dissociates in three steps:

1. H₃PO₄(aq) ⇌ H₂PO₄⁻(aq) + H⁺(aq) (Kₐ₁)
2. H₂PO₄⁻(aq) ⇌ HPO₄²⁻(aq) + H⁺(aq) (Kₐ₂)
3. HPO₄²⁻(aq) ⇌ PO₄³⁻(aq) + H⁺(aq) (Kₐ₃)

Typically, Kₐ₁ > Kₐ₂ > Kₐ₃, indicating that the first proton is the easiest to remove, and subsequent protons are increasingly difficult to dissociate. Understanding the stepwise dissociation of polyprotic acids is essential for accurately calculating the pH of their solutions and for predicting their behavior in chemical reactions.

Buffers and pH Stability

Buffers are solutions that resist changes in pH upon the addition of small amounts of acid or base. They typically consist of a weak acid and its conjugate base or a weak base and its conjugate acid.

The buffering capacity of a solution is highest when the concentrations of the weak acid and its conjugate base are equal. The pH of a buffer solution can be calculated using the Henderson-Hasselbalch equation:

pH = pKₐ + log([A⁻]/[HA])

Where:

• pKₐ is the negative logarithm of the acid dissociation constant.
• [A⁻] is the concentration of the conjugate base.
• [HA] is the concentration of the weak acid.

Buffers are essential in many biological and chemical systems, maintaining a stable pH environment necessary for proper function. Their ability to resist pH changes is critical in biological systems, chemical reactions, and analytical procedures.

Video: Percent Dissociation Formula: A Complete Guide

Play Video

So, there you have it! Everything you need to know to tackle those pesky percent dissociation formula problems. Hopefully, this guide has demystified the concept and given you the confidence to ace your next chemistry assignment. Good luck, and happy calculating!