Constant Velocity Explained: The Ultimate Viral Guide

Understanding motion is fundamental in physics, and constant velocity represents a crucial building block for more complex concepts. Newtonian mechanics, a cornerstone of classical physics, heavily relies on understanding objects moving at a constant velocity. The implications of maintaining a constant velocity are also evident in fields such as aerospace engineering, where designers must account for consistent motion during flight. Even MIT's physics curriculum emphasizes the importance of grasping constant velocity as a precursor to dynamics. To truly understand kinematics, mastering constant velocity principles, initially articulated and debated by figures like Galileo Galilei, is absolutely essential.

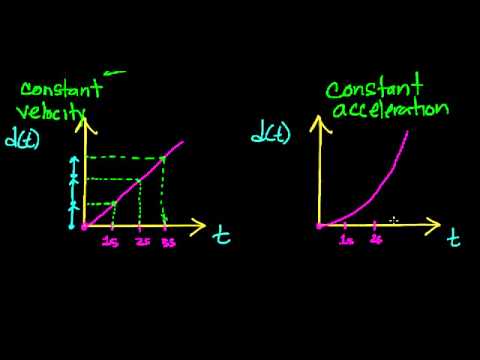
Image taken from the YouTube channel Timothy Palladino , from the video titled Constant Velocity compared to Constant Acceleration .

Imagine cruising down a seemingly endless highway.

Your speedometer is locked at a steady 65 mph.

Are you truly experiencing constant motion?

The answer, perhaps surprisingly, is likely no.

While your speed might be constant, your velocity isn't, especially if the road curves.

This seemingly simple scenario highlights the nuances of constant velocity.

It's a cornerstone of physics, yet often misunderstood.

This article serves as your comprehensive guide.

We'll demystify constant velocity and reveal its significance in both scientific principles and everyday life.

Challenging Perceptions of Motion

Our intuitive understanding of motion is often flawed.

We tend to equate constant speed with constant velocity.

However, physics demands a more precise definition.

True constant velocity implies movement in a straight line at a consistent speed.

Any deviation, be it a change in speed or direction, negates the "constant" nature of the velocity.

Consider a figure skater gliding across the ice in a straight line.

If they maintain their speed and direction, they're a good example.

However, the moment they curve into a spin, their velocity changes because they are changing direction.

Defining Constant Velocity: More Than Just Speed

In physics, velocity isn't merely about how fast something is moving.

It's a vector quantity.

This means it encompasses both magnitude (speed) and direction.

Therefore, constant velocity requires both a constant speed and a constant direction.

A car driving in a circle at a steady speed does not have constant velocity.

Its direction is continuously changing.

Thus, we must be precise in our understanding and application of constant velocity.

Why Constant Velocity Matters

Understanding constant velocity is fundamental to grasping more complex physics concepts.

It serves as the bedrock upon which we build our understanding of motion, forces, and energy.

It simplifies the often-complex equations of motion.

This allows us to isolate and analyze key variables without the added complications of acceleration.

Mastering constant velocity provides a stepping stone to understanding accelerated motion, projectile motion, and even orbital mechanics.

It is a crucial tool for simplifying problem-solving.

It allows us to focus on core principles.

A Roadmap to Understanding

This article will explore the concept of constant velocity from multiple angles.

First, we'll delve into the underlying physics, connecting it to inertia and Newton's First Law.

Next, we'll demonstrate how kinematics are simplified in scenarios with constant velocity.

Finally, we will showcase real-world examples.

These include transportation to space travel.

By the end of this journey, you will have a solid understanding of constant velocity.

You will appreciate its significance in both theoretical physics and the world around you.

Imagine cruising down a seemingly endless highway. Your speedometer is locked at a steady 65 mph. Are you truly experiencing constant motion? The answer, perhaps surprisingly, is likely no. While your speed might be constant, your velocity isn't, especially if the road curves. This seemingly simple scenario highlights the nuances of constant velocity. It's a cornerstone of physics, yet often misunderstood. This article serves as your comprehensive guide. We'll demystify constant velocity and reveal its significance in both scientific principles and everyday life. Challenging Perceptions of Motion Our intuitive understanding of motion is often flawed. We tend to equate constant speed with constant velocity. However, physics demands a more precise definition. True constant velocity implies movement in a straight line at a consistent speed. Any deviation, be it a change in speed or direction, negates the "constant" nature of the velocity. Consider a figure skater gliding across the ice in a straight line. If they maintain their speed and direction, they're a good example. However, the moment they curve into a spin, their velocity changes because they are changing direction. Defining Constant Velocity: More Than Just Speed In physics, velocity isn't merely about how fast something is moving. It's a vector quantity. This means it encompasses both magnitude (speed) and direction. Therefore, constant velocity requires both a constant speed and a constant direction. A car driving in a circle at a steady speed does not have constant velocity. Its direction is continuously changing. Thus, we must be precise in our language and understanding.

The previous section highlighted the distinction between speed and velocity, emphasizing that velocity is a vector quantity. Now, we delve deeper into the fundamental aspects of constant velocity. We will explore its relationship with motion, acceleration, displacement, and time. This exploration will further clarify what it truly means for velocity to remain constant.

Decoding the Fundamentals: What Makes Velocity Constant?

To truly grasp the concept of constant velocity, we must dissect its core components. It's not enough to simply say an object is moving at a steady pace. We need to understand how that pace is maintained and what implications it has for the object's motion.

Velocity as a Vector: Magnitude and Direction

Velocity, unlike speed, is a vector. This means it has both magnitude and direction. The magnitude of velocity is simply its speed (e.g., 60 mph). The direction specifies the path of motion (e.g., North, East, 30 degrees from horizontal).

For velocity to be constant, both its magnitude and direction must remain unchanged. A car traveling at a steady 60 mph due North possesses constant velocity. However, the same car, maintaining 60 mph while turning a corner, does not have constant velocity because its direction is changing.

Understanding this vector nature is critical to differentiating constant velocity from other forms of motion.

Constant Velocity vs. Non-Uniform Motion

Constant velocity is a special case of motion. It stands in contrast to non-uniform motion, where velocity is changing.

Non-uniform motion can manifest in several ways:

• Acceleration: The object is speeding up.
• Deceleration: The object is slowing down.
• Changing Direction: The object is turning, even if its speed is constant.

The defining characteristic of constant velocity is the absence of any of these changes. The object moves in a straight line at a steady pace, maintaining both its speed and direction. The unique characteristics of constant velocity greatly simplify our analysis of motion.

The Zero-Acceleration Imperative

Perhaps the most crucial aspect of constant velocity is its direct relationship with acceleration. Acceleration is defined as the rate of change of velocity. Thus, if velocity is constant, then its rate of change is zero.

In other words, constant velocity implies zero acceleration.

This is a fundamental principle in physics. Any force acting on an object will cause it to accelerate, thus changing its velocity. Only when the net force on an object is zero can it maintain constant velocity.

This relationship is expressed mathematically as:

a = 0

Where 'a' represents acceleration.

Displacement and Time: A Linear Relationship

With constant velocity, the relationship between displacement (change in position) and time becomes remarkably simple. Since the object is moving at a steady rate in a straight line, the displacement increases linearly with time.

This means that if you plot the object's position on a graph with time on the x-axis and displacement on the y-axis, you will obtain a straight line. The slope of this line represents the object's constant velocity.

This linear relationship provides a powerful tool for predicting an object's future position if we know its initial position, velocity, and the elapsed time.

The equation that expresses this is:

Δx = v * Δt

Where:

• Δx is the displacement
• v is the constant velocity
• Δt is the change in time.

Understanding the linear relationship between displacement and time is essential for solving constant velocity problems.

Thus, we must be precise in our definitions to truly grasp the essence of constant velocity. It's not just about what we see on the surface, but about the underlying principles that govern motion.

Let's delve deeper into these underlying principles and explore the physics that breathes life into the concept of constant velocity.

The Physics Behind Constant Velocity: Inertia and Newton's First Law

Constant velocity isn't just a descriptive term.

It's a consequence of fundamental physical laws.

Specifically, it is elegantly explained by Newton's First Law of Motion, often referred to as the Law of Inertia.

Newton's First Law: The Foundation of Constant Velocity

Newton's First Law states that an object at rest stays at rest and an object in motion stays in motion with the same speed and in the same direction unless acted upon by an unbalanced force.

This seemingly simple statement is profound.

It implies that constant velocity is the natural state of an object when no external forces interfere.

In other words, maintaining a constant speed in a straight line requires no force input; it simply happens due to inertia.

This might seem counterintuitive, especially in our everyday experiences.

We are constantly battling friction, air resistance, and other forces that tend to slow things down.

However, imagine a scenario where these forces are negligible, such as an object in deep space.

Inertia: The Resistance to Change

Inertia is the inherent tendency of an object to resist changes in its state of motion.

It is directly proportional to an object's mass.

A more massive object possesses greater inertia.

Meaning, it is harder to start moving, stop moving, or change its direction compared to a less massive one.

This resistance to change is what allows an object to maintain its constant velocity.

Without inertia, any tiny disturbance would cause an object to deviate from its path or change its speed.

Think of a bowling ball versus a soccer ball.

The bowling ball, with its greater mass, is far more resistant to changes in motion.

This means once you get that bowling ball rolling at a constant speed, it will take a lot more force to stop it.

The soccer ball with less mass will be far easier to stop.

The Role of External Forces: Disrupting Constant Velocity

While inertia allows an object to maintain constant velocity in the absence of external forces, the reality is that such idealized conditions rarely exist.

In our everyday world, external forces are ubiquitous, and they constantly act to disrupt constant velocity.

Two of the most common culprits are friction and air resistance.

Friction

Friction is a force that opposes motion between two surfaces in contact.

It acts in the opposite direction of the object's velocity, causing it to slow down over time.

A hockey puck sliding across the ice eventually comes to rest due to friction between the puck and the ice surface.

To maintain a constant velocity, a force equal in magnitude and opposite in direction to the frictional force must be applied.

Air Resistance

Air resistance, also known as drag, is a force that opposes the motion of an object through the air.

It depends on the object's speed, shape, and the density of the air.

A cyclist coasting on a level road will gradually slow down due to air resistance.

Again, maintaining constant velocity requires the cyclist to pedal, providing a force that counteracts the air resistance.

Real-World Implications

These examples illustrate that constant velocity is not an easily achieved state in our daily lives.

Maintaining it requires a constant balancing act between inertia and external forces.

A car moving at a constant speed on the highway needs its engine to constantly overcome friction and air resistance.

An airplane flying at a constant altitude and speed needs its engines to generate thrust equal to the drag force and maintain lift.

Understanding the interplay between inertia, external forces, and Newton's First Law is crucial for comprehending the dynamics of motion and predicting how objects will behave in various situations.

Thus, we must be precise in our definitions to truly grasp the essence of constant velocity. It's not just about what we see on the surface, but about the underlying principles that govern motion.

Let's delve deeper into these underlying principles and explore the physics that breathes life into the concept of constant velocity.

Constant Velocity in Action: Applying Kinematics

Constant velocity, while seemingly simple, has profound implications when applying the principles of kinematics. Kinematics, the branch of physics concerned with describing motion, becomes significantly streamlined when dealing with objects moving at a constant velocity. The absence of acceleration simplifies many equations, allowing for direct calculations of displacement, time, and velocity.

Simplifying Kinematic Equations

The power of constant velocity truly shines when we examine how it simplifies the standard kinematic equations. The general kinematic equations, which describe motion with constant acceleration, include terms for initial velocity, final velocity, acceleration, time, and displacement. However, when acceleration is zero, as it is in the case of constant velocity, several of these equations reduce to much simpler forms.

Consider the following general kinematic equation:

d = v₀t + (1/2)at²

Where:

• d = displacement
• v₀ = initial velocity
• t = time
• a = acceleration

When acceleration (a) is zero, this equation simplifies to:

d = v₀t

This equation allows us to directly calculate the displacement (d) of an object if we know its initial velocity (v₀) and the time (t) it travels. It is a testament to the elegance and simplicity that constant velocity brings to kinematic analysis.

Similarly, consider the equation that relates final velocity (v), initial velocity, acceleration, and time:

v = v₀ + at

Again, if acceleration (a) is zero, this equation reduces to:

v = v₀

This confirms that the final velocity is equal to the initial velocity, a defining characteristic of constant velocity.

Problem-Solving with Constant Velocity: Step-by-Step Examples

To further illustrate the practical applications of constant velocity, let's work through some example problems. These examples will demonstrate how to calculate velocity, displacement, and time in scenarios where acceleration is zero.

Example 1: Calculating Displacement

A train travels at a constant velocity of 80 m/s for 15 seconds. What is the displacement of the train?

Solution:

1. Identify knowns:
   • Velocity (v) = 80 m/s
   • Time (t) = 15 s
2. Identify unknown:
   • Displacement (d) = ?
3. Apply the simplified kinematic equation:
   • d = vt
4. Substitute values and solve:
   • d = (80 m/s) * (15 s) = 1200 m

Therefore, the displacement of the train is 1200 meters.

Example 2: Calculating Time

A cyclist rides at a constant velocity of 12 m/s and needs to travel 300 meters. How long will it take them?

Solution:

1. Identify knowns:
   • Velocity (v) = 12 m/s
   • Displacement (d) = 300 m
2. Identify unknown:
   • Time (t) = ?
3. Apply the simplified kinematic equation:
   • d = vt
4. Rearrange the equation to solve for time:
   • t = d/v
5. Substitute values and solve:
   • t = (300 m) / (12 m/s) = 25 s

Therefore, it will take the cyclist 25 seconds to travel 300 meters.

Example 3: Calculating Velocity

A car travels 500 meters in 20 seconds at a constant velocity. What is the velocity of the car?

Solution:

1. Identify knowns:
   • Displacement (d) = 500 m
   • Time (t) = 20 s
2. Identify unknown:
   • Velocity (v) = ?
3. Apply the simplified kinematic equation:
   • d = vt
4. Rearrange the equation to solve for velocity:
   • v = d/t
5. Substitute values and solve:
   • v = (500 m) / (20 s) = 25 m/s

Therefore, the velocity of the car is 25 m/s.

Visualizing Constant Velocity

Visual aids like diagrams and graphs are invaluable tools for understanding and representing constant velocity. A displacement-time graph for an object moving at constant velocity will always be a straight line. The slope of this line represents the velocity of the object. A steeper slope indicates a higher velocity, while a shallower slope indicates a lower velocity. If the slope is zero (a horizontal line), the object is at rest.

Conversely, a velocity-time graph for an object moving at constant velocity will be a horizontal line. The y-value of this line represents the constant velocity of the object. The area under this line represents the displacement of the object over a given time interval.

These graphical representations provide a powerful way to visualize and interpret the motion of objects moving at constant velocity. By understanding how these graphs relate to the kinematic equations, one can gain a deeper understanding of the concept and its applications.

Thus, we’ve seen how constant velocity simplifies the math, giving us clean and elegant equations. But where does this idealized concept show up in the world around us? Are there real-world scenarios where things actually move at a constant velocity? The answer, surprisingly, is yes, though with a few caveats.

Constant Velocity in the Real World: Everyday Examples

While perfectly constant velocity is an idealization, a useful tool for understanding more complex motion, many real-world scenarios approximate it closely enough to be valuable. From the familiar hum of a car on cruise control to the silent drift of objects in the vastness of space, constant velocity manifests itself in diverse and fascinating ways. Recognizing these instances allows us to apply our understanding of kinematics to predict and analyze motion around us.

Transportation: Maintaining a Steady Course

Transportation provides some of the most relatable examples of constant velocity, though, as with everything in the real world, it's crucial to consider the limitations.

Cars on Cruise Control: When a car is set to cruise control on a flat, straight highway, it attempts to maintain a constant speed. The engine adjusts automatically to counteract friction and air resistance, striving for a state where the net force is close to zero, thus keeping the velocity nearly constant. However, even with cruise control, slight variations in speed occur due to changes in road grade or wind conditions.

Airplanes in Flight: Similarly, an airplane flying at a constant altitude and constant speed on a straight path is also a good approximation of constant velocity. The pilots adjust the engine thrust to balance air resistance and maintain a steady course. Again, atmospheric turbulence and minor course corrections mean the velocity isn’t perfectly constant, but it’s often close enough for practical calculations.

Constant Velocity in Space: Inertia's Playground

Space, far from the influences of atmospheric drag and friction, provides a more pristine environment for observing constant velocity. Newton's First Law truly shines here.

Objects Moving Under Inertia: In the vacuum of space, once an object is set in motion, it tends to stay in motion at a constant velocity unless acted upon by an external force. This principle of inertia is fundamental to understanding how spacecraft navigate and how celestial bodies move.

Spacecraft Coasting Through Space: A spacecraft traveling between planets, after its initial propulsion phase, often spends a significant amount of time coasting at a constant velocity. Small course corrections are made periodically using thrusters, but for the vast majority of the journey, the spacecraft moves under its own inertia. This reliance on constant velocity minimizes fuel consumption and allows for efficient long-distance travel.

Other Engaging Examples

Beyond transportation and space, constant velocity concepts appear in a variety of other scenarios.

Assembly Lines: Consider an assembly line where objects move at a constant speed from one station to another. While the individual components being assembled may undergo complex movements, the overall motion of the conveyor belt approximates constant velocity. This ensures a consistent flow of products and efficient manufacturing processes.

Amusement Park Rides: Certain amusement park rides, like a Ferris wheel rotating at a constant speed, can also illustrate constant velocity, albeit with a twist. While the speed is constant, the direction is constantly changing, which means the velocity is not constant in the strictest sense. However, we can analyze the tangential speed as a constant value when considering the magnitude of the velocity at any given point.

Gliding on Ice: A skater gliding on a smooth, level ice surface after an initial push exemplifies constant velocity. With minimal friction to slow them down, the skater maintains their speed and direction, showcasing the power of inertia.

By recognizing constant velocity in these diverse contexts, we can appreciate its fundamental role in describing and predicting motion in the real world. While perfectly constant velocity is rare, these approximations provide valuable insights and allow us to apply the principles of kinematics to solve real-world problems.

Video: Constant Velocity Explained: The Ultimate Viral Guide

Play Video