Compressional Wave Definition: Sound & Properties

A compressional wave, also known as a longitudinal wave, is defined by its particle displacement being parallel to the direction of propagation, creating areas of compression and rarefaction. Sound, a common example of compressional waves, exhibits varying properties such as frequency and amplitude, which determine its pitch and loudness, respectively. The study of these waves is crucial in fields like seismology, where organizations like the United States Geological Survey (USGS) use compressional waves generated by earthquakes to understand Earth's internal structure. Analyzing compressional wave behavior often involves specialized tools, including oscilloscopes, which are used to visualize and measure wave characteristics, enabling precise analysis of their properties.

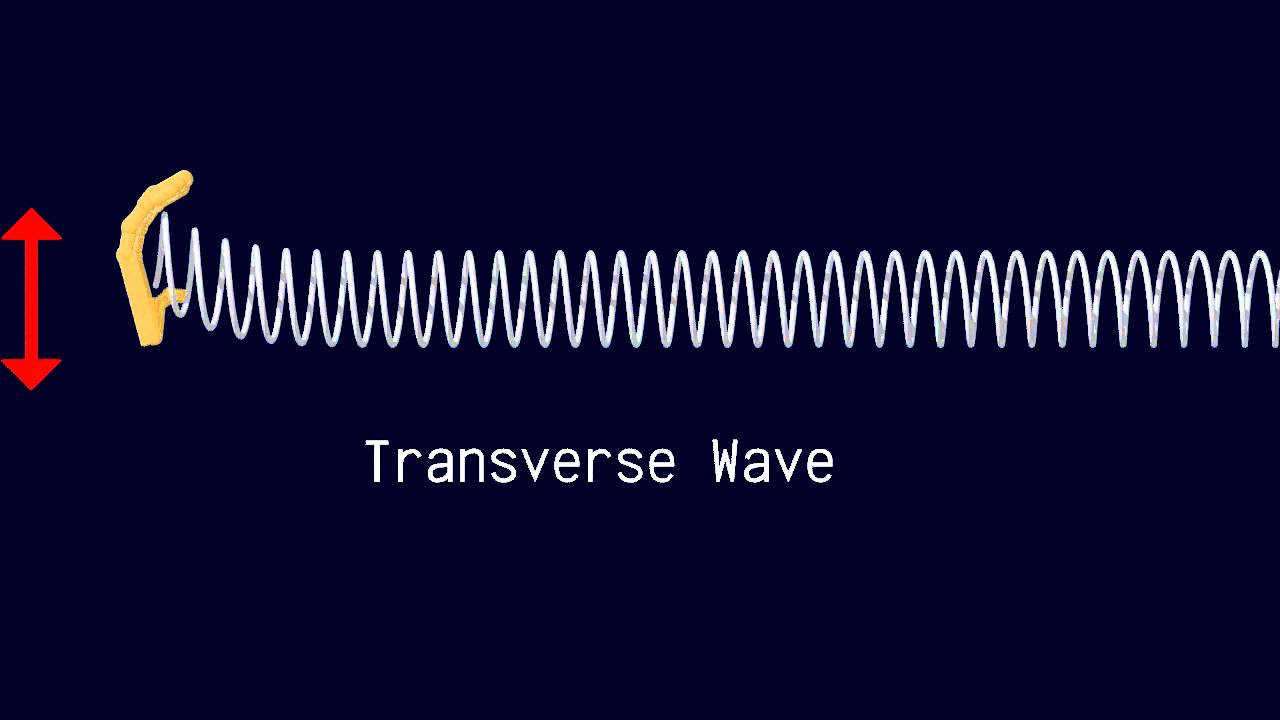
Image taken from the YouTube channel Animations for Physics and Astronomy , from the video titled Longitudinal and Transverse Waves .

Compressional Waves: A Fundamental Force Shaping Our World

Compressional waves, also recognized as longitudinal waves, constitute a fundamental aspect of wave physics, permeating both the natural world and technological applications.

These waves, unlike their transverse counterparts, are characterized by particle displacement that is parallel to the direction of wave propagation. This distinction is critical for understanding their unique properties and behavior.

Defining Compressional Waves: Longitudinal Motion

At their core, compressional waves are defined by their longitudinal motion. Imagine a Slinky being pushed and pulled along its length. The coils move back and forth in the same direction as the disturbance travels. This is analogous to how particles behave in a compressional wave.

In contrast, transverse waves, such as light waves or waves on a string, involve particle motion perpendicular to the direction of wave travel. This fundamental difference in particle motion dictates many of the distinguishing characteristics between these two wave types.

Acoustic Waves, Pressure Waves: Exploring Synonymous Terminology

The terms "pressure waves" and "acoustic waves" are frequently used interchangeably with "compressional waves," reflecting the underlying mechanism of wave propagation. Compressional waves propagate through a medium by creating regions of compression and rarefaction.

Compression refers to areas where particles are densely packed together, resulting in increased pressure. Rarefaction, conversely, describes regions where particles are more spread out, leading to decreased pressure.

These pressure variations are what we perceive as sound when they reach our ears. Therefore, the terms acoustic and pressure wave refer to compressional waves.

Ubiquitous Presence: From Earthquakes to Medical Imaging

Compressional waves are far from being an abstract concept; they are ubiquitous in our environment. They are at play in diverse scenarios ranging from catastrophic natural events to sophisticated technological applications.

Seismic P-waves, generated during earthquakes, are compressional waves that travel through the Earth's interior, providing valuable insights into its structure. The speed at which these compressional waves propagate is critical to detecting and classifying the magnitude of earthquakes.

Sound itself, the very medium through which we communicate and experience the world, is a compressional wave. Without compressional waves, there would be no transmission of music, speech, or any audible signals.

Furthermore, medical ultrasound relies on high-frequency compressional waves to create real-time images of internal organs, aiding in diagnosis and treatment. Sonar also uses compressional waves to navigate and map the ocean floor. These examples serve to illustrate the far-reaching impact of compressional waves across various scientific and technological domains.

Fundamental Principles: Understanding Wave Mechanics

Having established the essence of compressional waves, we now turn our attention to the underlying principles that govern their behavior. Grasping these fundamentals – encompassing wave characteristics, the influence of medium properties, and wave interactions – is crucial for a deeper understanding of compressional waves and their diverse applications.

Wave Characteristics: Defining the Nature of Compressional Waves

Compressional waves, like all waves, are characterized by several key parameters that dictate their behavior. Understanding these characteristics is essential for analyzing and manipulating these waves in various applications.

Wavelength (λ): The Spatial Extent of a Cycle

The wavelength (λ) is defined as the distance between two consecutive points in a wave that are in phase, such as the distance between two consecutive compressions or rarefactions. Wavelength is typically measured in meters (m) or its submultiples.

The wavelength has a profound impact on how a wave interacts with objects and its ability to propagate through different media. Shorter wavelengths tend to scatter more easily, while longer wavelengths can diffract around obstacles more effectively.

Frequency (f): The Temporal Rate of Oscillation

Frequency (f) refers to the number of complete wave cycles that pass a given point per unit time. It is measured in Hertz (Hz), where 1 Hz represents one cycle per second.

In the context of audible sound, frequency is directly related to the perception of pitch. Higher frequencies correspond to higher pitches, while lower frequencies correspond to lower pitches.

Amplitude: Gauging Wave Intensity and Energy

The amplitude of a compressional wave represents the maximum displacement of particles from their equilibrium position as the wave passes. Amplitude is related to the intensity and energy of the wave.

Higher amplitude waves carry more energy and are perceived as louder sounds or more intense pressure variations.

Speed of Sound (v): A Crucial Parameter

The speed of sound (v) is a critical parameter that describes how fast a compressional wave propagates through a medium. It's profoundly influenced by the properties of the medium itself.

The speed of sound is essential for calculations involving wave propagation, such as determining the distance to a sound source or designing acoustic systems.

Medium Properties: The Influence on Wave Propagation

The characteristics of the medium through which a compressional wave travels have a significant impact on its speed and behavior. Two key properties are elasticity and density.

Elasticity (Bulk Modulus): Resistance to Compression

Elasticity, specifically the bulk modulus (B), quantifies a medium's resistance to uniform compression.

A higher bulk modulus indicates that the medium is more resistant to compression, resulting in a higher speed of sound. Stiffer materials, therefore, generally transmit sound more rapidly.

Density (ρ): Mass per Unit Volume

Density (ρ), defined as mass per unit volume, plays an inverse role in determining the speed of sound. Higher density generally leads to a lower speed of sound.

This is because denser materials offer more inertia to the wave, hindering its propagation.

Wave Interactions: Reflection, Refraction, and Interference

Compressional waves exhibit various behaviors when they encounter boundaries or interact with other waves. These include reflection, refraction, and interference.

Reflection: Bouncing Off Boundaries

Reflection occurs when a compressional wave encounters a boundary between two media and a portion of the wave's energy is redirected back into the original medium.

The amount of reflection depends on the difference in acoustic impedance between the two media.

Refraction: Changing Direction

Refraction refers to the change in direction of a wave as it passes from one medium to another. This occurs because the speed of sound varies between the two media.

The angle of refraction is governed by Snell's law, which relates the angles of incidence and refraction to the speeds of sound in the two media.

Interference: Superposition of Waves

Interference occurs when two or more compressional waves overlap in the same region of space.

This can result in constructive interference, where the amplitudes of the waves add together, or destructive interference, where the amplitudes cancel each other out. These interference patterns are crucial in applications like noise cancellation.

Historical Context: Pioneering Figures in Wave Physics

Having established the essence of compressional waves, we now turn our attention to the underlying principles that govern their behavior. Grasping these fundamentals – encompassing wave characteristics, the influence of medium properties, and wave interactions – is crucial for a deeper understanding. However, it is equally essential to recognize the individuals whose insights paved the way for our current knowledge. Exploring the historical context reveals the evolution of understanding wave physics, lending a richer appreciation for the subject.

Key Contributors to the Understanding of Waves

The study of waves, including compressional waves, owes a debt to numerous brilliant minds. While a comprehensive list would be extensive, highlighting a few pivotal figures provides valuable perspective. These individuals, through their groundbreaking work, laid the foundation for modern acoustics and wave mechanics.

Isaac Newton's Foundation in Mechanics

Isaac Newton, a towering figure in scientific history, made substantial contributions that indirectly, yet profoundly, impacted our understanding of wave propagation. His laws of motion and universal gravitation provided the fundamental framework for classical mechanics, which is essential for describing wave phenomena.

Newton's meticulous work provided the bedrock upon which later scientists built their theories of sound and wave mechanics. While he did not directly focus on compressional waves as we understand them today, his work on mechanics was indispensable.

His emphasis on mathematical rigor and quantitative analysis set a new standard for scientific inquiry, influencing generations of physicists who followed. This rigorous approach was instrumental in developing accurate models and descriptions of wave behavior.

Christian Doppler and the Doppler Effect

Christian Doppler, an Austrian mathematician and physicist, is best known for his discovery of the Doppler Effect in 1842. This effect describes the change in frequency of a wave in relation to an observer who is moving relative to the wave source.

The Doppler Effect is particularly relevant to compressional waves, as it explains the perceived change in pitch of a sound as a source approaches or recedes. This phenomenon has far-reaching implications in various fields, from astronomy to medical imaging.

Doppler's initial observations were met with some skepticism, but they were later experimentally confirmed by Buys Ballot using acoustic waves. The effect has since become a cornerstone of wave physics and continues to be actively studied and applied in numerous disciplines. Its impact on our ability to understand the universe and develop advanced technologies is undeniable.

Propagation and Behavior: Delving Deeper into Compressional Wave Dynamics

Having established the essence of compressional waves, we now turn our attention to the underlying principles that govern their behavior. Grasping these fundamentals – encompassing wave characteristics, the influence of medium properties, and wave interactions – is crucial for a deeper understanding. This section, therefore, pivots to explore the nuances of compressional wave propagation. We will delve into the significant factors influencing how these waves travel.

Speed of Sound: The Guiding Factor

The speed of sound is not a universal constant. It is heavily dependent on the medium through which the wave propagates. Understanding this dependence is key to predicting and manipulating compressional wave behavior.

Mathematical Formulation: Unveiling the Relationship

The speed of sound (v) is mathematically defined as:

v = √(B/ρ)

Where:

• B represents the bulk modulus of the medium, which quantifies its resistance to uniform compression.
• ρ signifies the density of the medium, representing its mass per unit volume.

This equation reveals an important relationship: the speed of sound increases with the medium's resistance to compression and decreases with its density.

Medium Dependence: Illustrative Examples

The speed of sound exhibits significant variation across different media, directly correlating with their respective bulk moduli and densities.

• Air: In air, the speed of sound is relatively slow, approximately 343 meters per second at room temperature (20°C).

  This slower speed is attributable to air's low density and relatively low bulk modulus.

• Water: Water, being denser and less compressible than air, exhibits a significantly higher speed of sound.

  Sound travels through water at approximately 1480 meters per second.

• Solids: Solids, characterized by their high density and high bulk moduli, generally exhibit the highest speeds of sound.

  For example, sound can travel through steel at speeds approaching 5960 meters per second. This showcases the dramatic impact of medium properties.

Acoustic Properties: Impedance and Interfaces

Beyond the speed of sound, the acoustic properties of a medium play a crucial role in how compressional waves interact with their environment. Specifically, acoustic impedance governs the reflection and transmission of sound waves at interfaces between different media.

Acoustic Impedance: Defining the Opposition

Acoustic impedance (Z) is a measure of a medium's resistance to the passage of a sound wave. It is defined as the product of the medium's density (ρ) and the speed of sound within it (v):

Z = ρv

When a sound wave encounters an interface between two media with differing acoustic impedances, a portion of the wave is reflected back into the first medium, while the remainder is transmitted into the second. The amount of reflection and transmission depends on the magnitude of the impedance mismatch. A larger impedance difference results in a greater proportion of the wave being reflected.

Understanding the interplay between speed of sound and acoustic impedance is fundamental to mastering the behavior of compressional waves in complex environments. This knowledge becomes invaluable in diverse fields ranging from materials science to advanced acoustic engineering applications.

The Doppler Effect: Frequency Shifts in Motion

Having established the essence of compressional waves, we now turn our attention to the underlying principles that govern their behavior. Grasping these fundamentals – encompassing wave characteristics, the influence of medium properties, and wave interactions – is crucial for understanding the Doppler Effect. This section provides an in-depth explanation of this crucial phenomenon related to compressional waves. It explores the underlying principles and highlights its diverse applications.

Understanding the Doppler Effect

The Doppler Effect describes the change in frequency or wavelength of a wave in relation to an observer who is moving relative to the wave source. It's a phenomenon most readily observed with sound waves, but it applies to all waves, including electromagnetic waves like light. The effect arises from the compression or stretching of the wave as the source and observer approach or recede from one another.

Imagine an ambulance speeding towards you with its siren blaring. As it approaches, the sound of the siren seems higher pitched than it actually is. This is because the sound waves are compressed in front of the ambulance, effectively shortening their wavelength and increasing their frequency.

Conversely, as the ambulance passes and moves away, the pitch of the siren drops noticeably. In this case, the sound waves are stretched behind the ambulance, increasing their wavelength and decreasing their frequency. This perceived change in frequency is the essence of the Doppler Effect.

A Closer Look at the Physics

The frequency shift observed in the Doppler Effect is directly proportional to the relative velocity between the source and the observer. When the source and observer are approaching each other, the observed frequency f' is higher than the emitted frequency f.

The relationship can be expressed mathematically as:

f' = f (v + vo) / (v - vs)

Where:

• f' is the observed frequency.
• f is the emitted frequency.
• v is the speed of the wave in the medium.
• vo is the velocity of the observer relative to the medium (positive if moving towards the source, negative if moving away).
• vs is the velocity of the source relative to the medium (positive if moving towards the observer, negative if moving away).

This equation reveals that the magnitude of the frequency shift depends on the speeds of both the source and the observer relative to the medium through which the wave is traveling.

Applications of the Doppler Effect

The Doppler Effect is not merely a theoretical curiosity; it has a plethora of practical applications that have revolutionized various fields.

Radar Technology

Radar (Radio Detection and Ranging) utilizes the Doppler Effect to determine the speed of objects, such as vehicles or aircraft. By emitting radio waves and analyzing the frequency shift in the reflected waves, radar systems can accurately measure the velocity of a target.

This is instrumental in air traffic control, weather forecasting, and law enforcement.

Astronomy

In astronomy, the Doppler Effect plays a crucial role in determining the motion of stars and galaxies. By analyzing the spectral lines of light emitted by celestial objects, astronomers can infer whether they are moving towards or away from Earth.

This phenomenon, known as redshift (for objects moving away) and blueshift (for objects moving towards), provides invaluable information about the expansion of the universe and the dynamics of galaxies.

Medical Diagnostics

Doppler ultrasound is a non-invasive medical imaging technique that uses the Doppler Effect to assess blood flow in arteries and veins. By measuring the frequency shift of ultrasound waves reflected from blood cells, physicians can detect blockages, aneurysms, and other vascular abnormalities.

This technique is widely used in cardiology, obstetrics, and vascular surgery.

Other Applications

Beyond these prominent examples, the Doppler Effect finds application in various other domains, including:

• Speed Guns: Measuring the speed of vehicles in sports or by law enforcement.

• Flow Measurement: Determining the flow rate of fluids in pipes or channels.

• Vibration Analysis: Analyzing the vibration patterns of mechanical systems.

The Doppler Effect stands as a powerful example of how understanding fundamental wave phenomena can lead to innovative technologies with profound implications across diverse fields. Its continued application promises further advancements in science, medicine, and engineering.

Applications and Technologies: Harnessing Compressional Waves

Having established the essence of compressional waves, we now turn our attention to the diverse applications that leverage their unique properties. From the depths of the ocean to the intricacies of medical diagnostics, compressional waves are harnessed in a myriad of technologies, profoundly impacting various fields.

This section explores several key applications, underscoring the practical significance and transformative potential of these ubiquitous waves.

Sound Navigation and Ranging (Sonar)

Sonar, an acronym for Sound Navigation and Ranging, represents a pivotal application of compressional wave technology. Primarily utilized for underwater object detection, navigation, and mapping, sonar systems exploit the propagation characteristics of acoustic waves in aquatic environments.

Active Sonar: Echoes in the Deep

Active sonar employs a straightforward yet effective principle: the transmission and reception of compressional waves. A transducer emits a precisely controlled pulse of sound into the water, and the system then listens for echoes reflected off submerged objects or the seabed.

By meticulously analyzing the time delay, frequency shift (due to the Doppler effect), and intensity of the returning echoes, sonar systems can determine the distance, speed, size, and composition of targets.

The accuracy of active sonar is influenced by several factors, including water temperature, salinity, and pressure, which affect the speed of sound. Sophisticated algorithms are employed to compensate for these variations, ensuring reliable performance across diverse oceanic conditions. While powerful, active sonar is subject to potential detection, thereby, potentially endangering its operator in some circumstances.

Medical Applications: Imaging the Unseen

Compressional waves have revolutionized medical diagnostics, offering non-invasive methods for visualizing internal structures and monitoring physiological processes. Ultrasound imaging, in particular, stands out as a versatile and widely adopted technique.

Ultrasound Imaging: A Window into the Body

Ultrasound imaging utilizes high-frequency sound waves, typically ranging from 2 to 18 MHz, to generate real-time images of soft tissues, organs, and blood flow. A handheld transducer emits sound waves that penetrate the body, and the returning echoes are processed to create detailed anatomical images.

The principles underlying ultrasound imaging are similar to those of sonar, but with a crucial distinction: the frequencies employed are significantly higher. These high-frequency waves provide superior resolution, enabling the visualization of intricate details within the human body.

Moreover, the safety profile of ultrasound is a key advantage. Unlike X-rays, ultrasound does not involve ionizing radiation, making it safe for repeated use and suitable for imaging pregnant women and children.

Different modes of ultrasound imaging exist to visualize certain medical conditions. For instance, Doppler ultrasound measures the change in frequency of the sound waves as they bounce off moving objects, such as blood cells, which can be used to measure the speed and direction of blood flow. This is critical in diagnosing conditions like deep vein thrombosis or arterial stenosis.

Fields of Study: Exploring the Realm of Acoustics

Having explored the intricacies of compressional waves and their applications, it becomes essential to contextualize this knowledge within the broader scientific discipline that governs these phenomena. This section serves as an introduction to the multifaceted field of acoustics, which is a cornerstone of understanding how sound is generated, propagates, and interacts with its environment.

Acoustics Defined: A Multidisciplinary Science

Acoustics, in its broadest sense, is the science concerned with the production, control, transmission, reception, and effects of sound. It's an inherently interdisciplinary field, drawing upon principles from physics, engineering, psychology, and even the arts.

Its scope extends far beyond the audible range, encompassing infrasound (below human hearing) and ultrasound (above human hearing), both of which play critical roles in various technologies and natural processes.

Subdisciplines Within Acoustics

The breadth of acoustics necessitates specialization, leading to the development of numerous subdisciplines, each focusing on specific aspects of sound and vibration. These include:

Architectural Acoustics

Architectural acoustics deals with the control of sound within buildings and the design of spaces to optimize sound quality. This involves considerations of reverberation time, sound absorption, and noise isolation to create environments suitable for speech, music, or other activities. Effective architectural acoustics are crucial for concert halls, classrooms, and even residential buildings.

Underwater Acoustics

Underwater acoustics focuses on the propagation and behavior of sound in the marine environment. This is essential for sonar technology, marine mammal communication studies, and understanding the impact of human activities on ocean life. Understanding the physics of sound in water is crucial for applications as diverse as submarine detection and seismic surveying.

Psychoacoustics

Psychoacoustics investigates the psychological and physiological responses to sound. It explores how humans perceive loudness, pitch, timbre, and other auditory attributes. This field is vital for the design of audio equipment, the development of hearing aids, and understanding the effects of noise on human health. It bridges the gap between the physical properties of sound and our subjective experience of it.

Noise Control

Noise control engineering focuses on reducing unwanted sound in various environments, from industrial settings to urban areas. It involves the application of various techniques, such as sound barriers, vibration damping, and active noise cancellation, to mitigate the harmful effects of noise pollution.

Musical Acoustics

Musical acoustics delves into the physics of musical instruments and the perception of music. It examines the sound production mechanisms of different instruments, the acoustics of concert halls, and the principles of musical harmony and tuning. This field provides a scientific basis for understanding the art of music.

The Importance of Acoustics

The field of acoustics plays a vital role in countless aspects of modern life. From designing quieter homes to developing advanced medical imaging techniques, the principles of acoustics are essential for solving real-world problems and improving our quality of life. Acoustical knowledge is fundamental to engineering, medicine, and the arts.

Professional Organizations: Advancing Acoustical Knowledge

Having explored the intricacies of compressional waves and their applications, it becomes essential to contextualize this knowledge within the broader scientific discipline that governs these phenomena.

This section highlights the critical role that professional organizations play in fostering research, promoting education, and ultimately, advancing our collective understanding of acoustics. These organizations serve as hubs for collaboration, knowledge dissemination, and the setting of standards within the field.

The Acoustical Society of America (ASA): A Cornerstone of Acoustical Research

The Acoustical Society of America (ASA) stands as one of the preeminent organizations dedicated to the advancement of acoustics.

Founded in 1929, the ASA's mission is multifaceted. Its primary goals include generating, disseminating, and promoting knowledge and practical applications of acoustics.

The ASA achieves these goals through a variety of activities.

Dissemination of Knowledge: Publications and Meetings

The ASA is a leading publisher of peer-reviewed journals and conference proceedings. These publications, such as the Journal of the Acoustical Society of America (JASA), serve as essential resources for researchers and practitioners alike.

JASA presents cutting-edge research across all areas of acoustics.

The ASA also holds bi-annual meetings.

These meetings provide a forum for researchers, engineers, and students to present their work, exchange ideas, and network with colleagues.

Fostering Education: Educational Initiatives

Recognizing the importance of nurturing the next generation of acousticians, the ASA actively supports educational initiatives.

This support includes student scholarships, grants, and outreach programs. These programs aim to inspire interest in acoustics among students at all levels.

The ASA also develops educational materials and resources for educators.

Setting Standards: Standards Development

The ASA plays a vital role in the development of acoustical standards. These standards are used in a wide range of industries.

They contribute to the safety, quality, and performance of products and systems.

These include noise measurement, hearing protection, and underwater acoustics.

Other Important Professional Acoustical Organizations

While the ASA is a prominent example, other organizations also contribute significantly to the field.

These include the Institute of Noise Control Engineering (INCE-USA) and various regional and international acoustical societies.

Each organization caters to specific areas within acoustics and offers unique resources for professionals and researchers.

The collective efforts of these organizations are indispensable for advancing the field of acoustics and ensuring its continued growth and relevance in addressing societal challenges.

Video: Compressional Wave Definition: Sound & Properties

Play Video

So, next time you're listening to music or just chatting with a friend, remember that you're experiencing the power of a compressional wave – specifically, the compressional wave definition in action, carrying sound through the air. Pretty cool, huh?