Circumference vs Perimeter: Difference & Uses

The fundamental distinction between circumference and perimeter lies in the shapes to which they apply: circumference specifically measures the distance around a circle, a concept extensively explored in Euclidean geometry, whereas perimeter is used for polygons, shapes with straight sides like those studied by Archimedes. Calculating the circumference involves mathematical constants, such as pi (π), whose approximate value is 3.14159, while determining the perimeter involves summing the lengths of all sides, a skill taught early in educational curricula, from institutions like Khan Academy, to build a solid foundation in geometry; thus, understanding the nuances of circumference vs perimeter is crucial for various applications ranging from basic construction to advanced engineering designs.

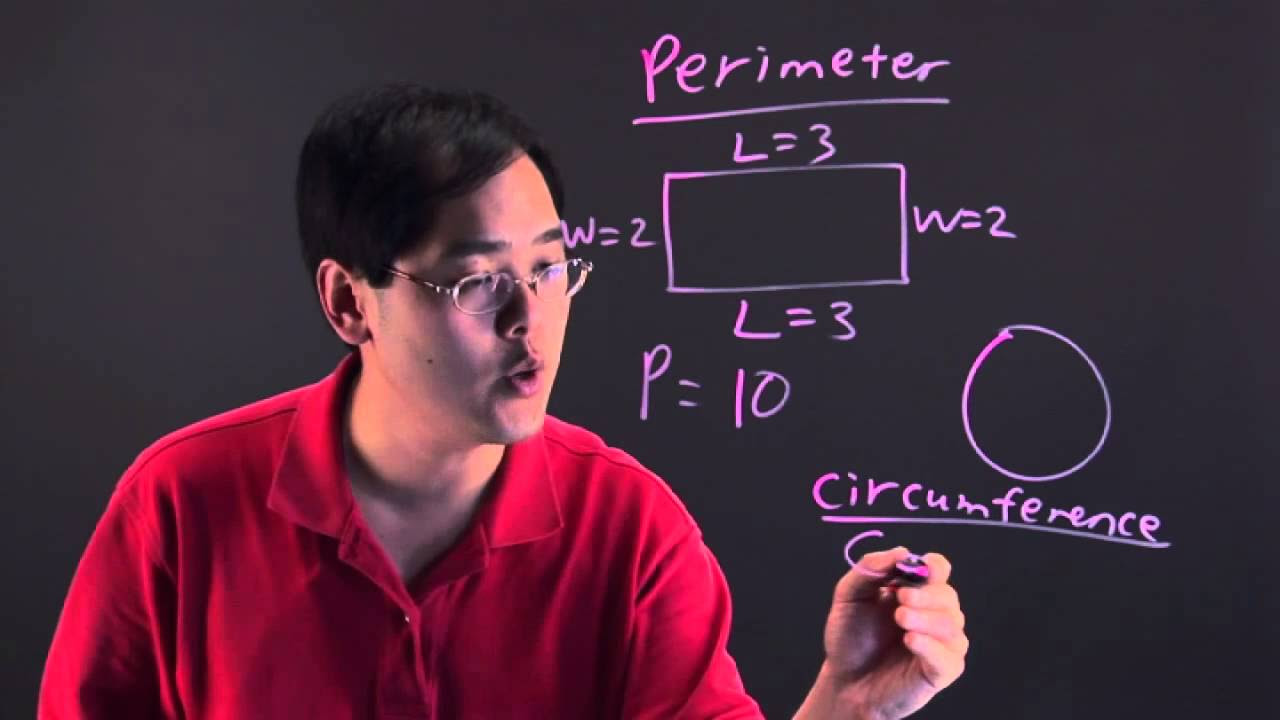
Image taken from the YouTube channel eHowEducation , from the video titled How to Do Perimeter & Circumference .

This section aims to introduce the foundational concepts of perimeter and circumference, clarifying their distinctions and highlighting their significance. These measurements are crucial in numerous fields, impacting how we design, build, and understand the world around us. We will also provide a general overview of the geometric shapes pertinent to these calculations.

Defining Perimeter and Circumference

Perimeter and circumference, while related, apply to different geometric figures. Perimeter refers to the total distance around any two-dimensional shape. It is found by summing the lengths of all the sides of the shape.

Circumference, on the other hand, is a specialized term. It specifically denotes the perimeter of a circle. It represents the distance around the circular boundary.

Understanding this distinction is the first step in mastering geometric measurements.

Importance and Applications

Perimeter and circumference are not mere theoretical concepts. They have profound practical applications across diverse fields.

In engineering, these measurements are critical for calculating material requirements, designing structural components, and ensuring dimensional accuracy.

In construction, perimeter informs the amount of fencing needed for a property. It also helps determine the length of baseboards for a room. Circumference dictates the amount of piping required for a circular tank.

Design also relies heavily on these concepts. Architects use perimeter to plan building layouts. Fashion designers use circumference to create garments that fit accurately.

From planning a garden to designing a spacecraft, perimeter and circumference calculations are indispensable.

Overview of Geometric Shapes

The calculation of perimeter and circumference is relevant to a variety of geometric shapes. Some common shapes include:

• Squares: Four equal sides and four right angles.
• Rectangles: Four sides with opposite sides equal and four right angles.
• Triangles: Three sides.
• Polygons: Any closed shape with straight sides.
• Circles: A shape with all points equidistant from a central point.

Each shape has its unique properties that influence how its perimeter or circumference is calculated. Understanding these properties is key to accurate measurement and application.

Foundational Geometric Concepts: Building Blocks for Calculation

This section aims to introduce the foundational concepts of perimeter and circumference, clarifying their distinctions and highlighting their significance. These measurements are crucial in numerous fields, impacting how we design, build, and understand the world around us. We will also provide a general overview of the geometric shapes pertinent to these calculations.

The Circle: Definition and Key Properties

The circle, a fundamental shape in geometry, is defined as the set of all points in a plane that are equidistant from a central point. This central point is known as the center of the circle.

A critical property of the circle is the constant ratio between its circumference (the distance around the circle) and its diameter (the distance across the circle through the center). This ratio is represented by the mathematical constant, Pi (π).

Radius and Diameter: Defining Circular Dimensions

The Radius

The radius is the distance from the center of the circle to any point on its edge. It's half the length of the diameter and is fundamental in calculating the circumference.

Knowing the radius allows for precise determination of a circle’s size and is crucial in various applications, from engineering to design.

The Diameter

The diameter, on the other hand, is a line segment that passes through the center of the circle and has endpoints on the circle's circumference. The diameter is equal to twice the radius.

The relationship between the radius and diameter provides flexibility in circumference calculations, depending on the available measurement.

Line Segments: Building Blocks of Polygons

A line segment is a straight path connecting two distinct endpoints. It’s a foundational element in geometry, particularly for constructing polygons.

Polygons, such as squares, rectangles, and triangles, are formed by connecting line segments to create closed, two-dimensional shapes. These line segments are essential to understanding perimeter.

The perimeter of a polygon is simply the sum of the lengths of its constituent line segments.

Pi (π): The Constant of Circumference

Pi (π) is a mathematical constant that represents the ratio of a circle's circumference to its diameter. It is an irrational number, meaning its decimal representation neither terminates nor repeats.

The approximate value of Pi is 3.14159. Pi is indispensable in calculating the circumference of a circle, using the formulas C = πd or C = 2πr.

Its existence highlights a universal property inherent to all circles.

Geometric Shape Examples and Perimeter Calculations

To solidify understanding, let’s look at a few examples.

Calculating the Perimeter of a Square

A square is a four-sided polygon where all sides are equal in length and all angles are 90 degrees.

To find its perimeter, simply add the length of one side four times. For example, if a square has a side length of 5 cm, its perimeter is 4

**5 cm = 20 cm.

Calculating the Perimeter of a Rectangle

A rectangle is a four-sided polygon with two pairs of equal-length sides and four 90-degree angles.

To calculate the perimeter, add the lengths of all four sides. If a rectangle has a length of 8 cm and a width of 3 cm, its perimeter is 2** (8 cm + 3 cm) = 22 cm.

Calculating the Perimeter of a Triangle

A triangle is a three-sided polygon. There are various types of triangles, including equilateral (all sides equal), isosceles (two sides equal), and scalene (no sides equal).

The perimeter of any triangle is found by adding the lengths of its three sides. For instance, if a triangle has sides of 4 cm, 6 cm, and 7 cm, its perimeter is 4 cm + 6 cm + 7 cm = 17 cm.

Calculating the Perimeter of a Polygon

A polygon is a closed, two-dimensional shape made up of straight line segments. Polygons can be regular (all sides and angles equal) or irregular (sides and angles not equal).

To calculate the perimeter of any polygon, simply add up the lengths of all its sides. This applies whether the polygon is a regular hexagon or an irregular pentagon.

Mathematical Principles: Formulas and Units of Measurement

Building upon our understanding of geometric shapes, we now transition to the mathematical tools that allow us to quantify their dimensions. This section will dissect the fundamental formulas for calculating circumference and perimeter, providing clarity on their application. We will also explore the standard units of measurement used in these calculations, bridging the gap between abstract formulas and practical application.

The Essence of Circumference: Unveiling the Formula

The circumference, a critical attribute of circles, is defined precisely by a succinct formula that links the circle's diameter or radius to its outer boundary. The formula, C = πd = 2πr, encapsulates the relationship between the circumference (C), the diameter (d), the radius (r), and the mathematical constant Pi (π).

Here, π represents the ratio of a circle's circumference to its diameter, an irrational number with an approximate value of 3.14159. The formula C = πd states that the circumference is equal to Pi times the diameter.

Equivalently, since the diameter is twice the radius (d = 2r), the formula can also be expressed as C = 2πr, showing that the circumference is also equal to two times Pi times the radius. Understanding these relationships is vital for accurate calculations and practical applications.

Demystifying Perimeter: Summing the Sides

Unlike the specialized formula for circles, calculating the perimeter of polygons follows a more direct approach. The perimeter of any polygon, regardless of its shape or the number of its sides, is simply the sum of the lengths of all its sides.

This principle holds true for triangles, squares, rectangles, and more complex multi-sided figures. To find the perimeter, one must measure the length of each side and add them together. This straightforward method is universally applicable to all polygonal shapes.

Units of Measurement: Standardizing Dimensions

Accurate measurement relies on standardized units, ensuring consistency and clarity in calculations. Two primary systems of measurement, the metric and imperial systems, are commonly used.

Metric System: Precision in Decimals

The metric system, widely adopted around the world, employs units such as centimeters (cm) and meters (m). A centimeter is one-hundredth of a meter, making conversions straightforward due to the decimal-based structure.

For example, measuring a small object might be done in centimeters, while measuring the dimensions of a room would typically be done in meters. The metric system’s decimal-based nature simplifies calculations and conversions, enhancing its utility in scientific and engineering applications.

Imperial System: Familiarity in Fractions

The imperial system, predominantly used in the United States, uses units such as inches (in) and feet (ft). An inch is a unit of length, and twelve inches make up a foot.

While conversions within the imperial system can be more complex due to their fractional relationships, familiarity with these units is essential in certain contexts. Measuring smaller items might be done in inches, while larger dimensions might be measured in feet.

Navigating Measurement Systems: Metric vs. Imperial

The choice between metric and imperial units often depends on regional standards or the specific field of application. While the metric system offers ease of use with its decimal base, the imperial system remains relevant in certain industries and regions.

Understanding both systems and their conversions is essential for professionals who work across different contexts or collaborate internationally. This knowledge ensures accurate communication and consistent results in any project involving measurements.

Tools and Techniques for Measurement: Practical Approaches

Building upon our understanding of geometric shapes, we now transition to the practical tools that allow us to quantify their dimensions. This section will dissect the instruments and methods used to accurately measure perimeter and circumference, providing clarity on their applications. We will explore rulers, measuring tapes, compasses, calculators, and online calculators, examining their strengths and limitations in various measurement scenarios.

The Humble Ruler: Precision in Straight Lines

The ruler, a staple of any measurement toolkit, excels at measuring straight lines. In perimeter calculations, it proves invaluable for shapes with clearly defined sides, such as squares, rectangles, and triangles.

To use a ruler effectively, align the zero mark with one endpoint of the line segment you wish to measure. Read the measurement at the other endpoint, ensuring your eye is directly above the ruler to avoid parallax error.

For more complex shapes, divide the perimeter into measurable straight segments and sum the individual lengths. While simple in concept, the ruler’s accuracy depends on the precision of its markings and the user's careful alignment.

Measuring Tapes: Conquering Irregularity

Measuring tapes offer flexibility when dealing with irregular shapes or longer distances. Unlike rulers, which are rigid, measuring tapes can conform to curves and contours, making them suitable for measuring the perimeter of objects with non-straight sides.

When using a measuring tape, ensure it is taut and follows the shape's outline closely. Read the measurement at the point where the tape completes the perimeter, being mindful of any slack that could introduce error.

Measuring tapes are particularly useful in real-world scenarios like landscaping, where garden beds and pathways rarely follow perfect geometric forms.

The Compass: Defining Circular Boundaries

While not directly used to measure circumference in the same way as a ruler or tape, the compass plays a crucial role in defining circles, the foundation of circumference. A compass allows for the precise creation of a circle, ensuring that every point on the circumference is equidistant from the center.

By setting the compass to a specific radius, you define the dimensions needed for the circumference calculation (C = 2πr).

The compass, therefore, acts as a tool for establishing the geometric parameters necessary for determining circumference through calculation.

Calculators: Bridging Complexity and Precision

Calculators are indispensable tools for simplifying complex calculations involving Pi (π) and larger numbers. When calculating circumference, especially with precise radius or diameter measurements, a calculator ensures accuracy and efficiency.

Similarly, when dealing with polygons with numerous sides or non-integer side lengths, a calculator can quickly sum the individual measurements to determine the total perimeter.

The calculator minimizes the risk of human error and expedites the calculation process.

Online Calculators: Streamlining Perimeter and Circumference

Online calculators offer a convenient alternative for perimeter and circumference calculations, particularly when dealing with complex shapes or multiple measurements. These tools typically require inputting the relevant dimensions, such as side lengths for polygons or radius/diameter for circles.

They then automatically perform the calculations, providing instant results.

Many online calculators also offer specialized functions for specific shapes, such as ellipses or irregular polygons, further simplifying the measurement process. While convenient, it's crucial to verify the calculator's accuracy and understand the underlying formulas to ensure reliable results.

Real-World Applications: Where Perimeter and Circumference Matter

Building upon our understanding of geometric shapes, we now transition to the practical tools that allow us to quantify their dimensions. This section will dissect the instruments and methods used to accurately measure perimeter and circumference, providing clarity on their applications. We will delve into how these fundamental concepts manifest in various sectors, demonstrating their crucial role in ensuring accuracy, efficiency, and safety.

Engineering: Precision in Design

In engineering, the precise calculation of perimeter and circumference is not merely an academic exercise; it's a foundational necessity. Structural integrity, mechanical efficiency, and design accuracy all hinge on these calculations.

Consider, for instance, the design of a bridge. The circumference of support pillars directly impacts their load-bearing capacity. The perimeter of a structural component dictates the distribution of stress.

Mechanical engineers rely heavily on circumference to calculate the speed and efficiency of rotating parts, ensuring optimal performance and minimizing wear and tear. Any deviation from accurate measurements can lead to catastrophic failures.

Construction: Material Optimization

The construction industry is another arena where perimeter and circumference reign supreme. Estimating material requirements—from fencing and flooring to roofing and piping—requires meticulous calculation of perimeters and circumferences.

For example, determining the amount of fencing needed for a property necessitates accurate perimeter measurements. Similarly, calculating the flooring required for a circular room depends directly on its circumference.

In roofing, the perimeter of a building determines the amount of edge flashing needed. Accurate calculations translate into cost savings by preventing material waste and ensuring projects stay within budget.

Piping and HVAC Systems

Perimeter and circumference are also vital when calculating the amount of insulation needed for pipes in plumbing and HVAC systems. Properly insulating pipes with the correct surface area, which is derived from circumference, prevents heat loss or gain, boosting energy efficiency and minimizing operational costs.

Landscaping: Defining Spaces Beautifully

Landscaping design benefits significantly from the application of perimeter and circumference. Defining garden beds, pathways, and other landscape features requires precise measurements to ensure aesthetic appeal and functional design.

The perimeter of a flower bed dictates the amount of edging required. The circumference of a circular patio determines the quantity of paving stones needed.

Moreover, these calculations play a crucial role in irrigation system design, ensuring uniform water distribution by determining the optimal placement and length of sprinkler lines. Accurate measurements in landscaping create visually pleasing and practical outdoor spaces.

Education: Foundational Knowledge

Textbooks and educational curricula emphasize geometry, including perimeter and circumference, as fundamental building blocks for mathematical literacy. These concepts are not merely abstract ideas; they are practical tools that empower students to understand and interact with the world around them.

Understanding perimeter and circumference lays the groundwork for more advanced mathematical concepts, such as area, volume, and trigonometry. It equips students with critical thinking and problem-solving skills that are applicable across various disciplines.

Video: Circumference vs Perimeter: Difference & Uses

Play Video

So, whether you're fencing a yard (perimeter!) or figuring out how much trim you need for a circular window (circumference!), understanding the difference between circumference vs perimeter is pretty handy. Hopefully, this clears up any confusion and helps you tackle those real-world geometry problems with a bit more confidence!